A survey was conducted to determine the popularity of 3 food

Bunuel

What is the difference between these two equations?

#1) Total = Group 1 + Group 2 + Group 3 - (sum of all 2 or more overlaps) + (in all 3 groups) + none.

#2) Total = Group 1 + Group 2 + Group 3 - (sum of members in EXACTLY 2 groups) - 2(All three groups) + none

The first equation was used on the problem above and I have seen the second equation used in this question "A survey was conducted to find out how many people in a housing colony of 144 residents could swim, dance and drive a car...."

Thanks

Check ADVANCED OVERLAPPING SETS PROBLEMS: https://gmatclub.com/forum/advanced-over ... 44260.html
_________________

Hi Bunuel request you to assist me

Only Pizza = P - (P and H + P and T - All 3) = 48 - (28 + 40 - 25) = 5;
While solving for Only one food.

If 28 (Pizza and Hoagies) Includes the G part i.e all three
and 40 (Pizza and Tacos) also includes the G part

Aren't we adding G 2 times and subtracting only once?

To further simply my query:

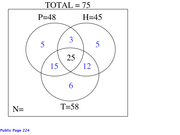
in the question it says 28 people like pizza and hoagies; however, the 28 people also include people who like pizza, hoagies AND tacos. Similarly for 40 question says 40 like Pizza and Hoagies; however, the 40 also includes people who like tacos.

When we add the two, i.e P and H + P and T don't we end up adding all three portion twice and subtract it only once?

Please help

How the stats are presented here doesnt make sense to me. I dont get it.

Consider my easier distribution of answers:

Only 10 students:

6 like pizza
4 like hogies
7 like tacos

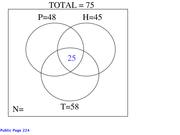
2 like all three
1 like pizza and hogies
1 like hogies and tacos
1 like pizza and tacos

6+4+7 = 17 "likes"

To get the number of students we take 17 - 2*2 - 1*3 = 10

Number of students = Total likes - 2*(all three) - 1*(two likes)


However, for the problem presented here this is not possible. Can someone please clarify why this doesnt work?

48 + 45 + 58 = 151
151 - 2*25 - 1*(28+37+40) = -4

So far so good. I got -4 because four students didnt like any of the foods.


Now, here is the issue:

To get those who only liked one of the foods, the logic fails:

In my own example I would just take PIZZA - ALLTHREE - PIZZAHOGIES - PIZZATACOS = ONLYPIZZA

In this illogical question it SHOULD be 48 - 25 - 28 - 40 = Hell lot of minus.


My question therefore is: how do these people even present data? Now I get what you all did here, subtracting 25 from both 28 and 40, but this really should not be necessary if the data was presented in a logical sense.

What I mean is: The ALLTHREE category should not be counted in the ONLYTWO categories. I really dont get why this is the case here? Or put it this way: why, by default, are the categories that consist of a pair of foods not considered ONLYTWO categories, but rather ATLEASTTWO? How do we know when the categories are considered ONLYTWOs and ATLEASTTWOS, respectively?


Edit: Since I found Bunuels' thread on Advanced overlapping sets, I know better. Thanks!

Has this been addressed in the TTP course? I too came across this type of questions and never recalled seeing that equation in TTP.

What is the different between "Unique members" vs "Total"?

Response:

In the formulas, “Unique Members” and “Total” refer to the same quantity. The former emphasises that adding the number of elements in groups A, B, and C does not necessarily give us the total number of elements, as the elements in overlaps will be counted multiple times.

In the TTP course, we teach the following two formulas:

Total # of Unique Elements = # in Group A only + # in Group B only + # in Group C only - # in Double Overlaps Only + # in Triple Overlap + # in Neither

Total # of Unique Elements = # in (Group A) + # in (Group B) + # in (Group C) - # in (Groups of Exactly Two) - 2[# in (Group of Exactly Three)] + # in (Neither)

There is also the formula Total # of Unique Elements = A + B + C − (sum of 2−group overlaps) + (all three) + Neither. The difference between this formula and the above formulas is that you can add the number of elements that belong to two or three groups directly, whereas in the above formulas you first have to subtract the number of elements of “Group of Exactly Three” each time before adding them. Using this formula might save you a few seconds, but we don’t think memorizing a third formula is worth the gain.
_________________

GMATNinja Just wanted to check, how would you feel about me sharing the method you (Harry) taught during the 24-hour stream, for tackling this question?

Thank you so much for asking, Vubar! Since we shared it publicly in a video, it's absolutely no problem to post it here, especially if it includes a shoutout to Harry.

And thank you for sticking with the whole marathon, Vubar! It meant a lot to me.
_________________

So it turns out this question doesn't need the whole method that Harry from GMAT Ninja taught (the better Harry ), so if you want to see the whole method, you can find it here: https://youtu.be/FJa6Dz5p1Ko?t=23573

Using the table he suggests, this question becomes very simple. Absolutely watch the video linked above (and if you can, please do support the cause they were fundraising for).

To sum up their method, I'd break it down into a few steps:
1a. Identify how many groups you have, with memberships that are overlapping (e.g., in this case, the number of foods liked by any individual).
1b. Label a column "# groups" with the word groups replaced with whatever title is appropriate.
1c. Fill in the numbers for the groups with the information you know from the question.

2a. Make a second column for the number of members (e.g., in this case, the # of students)
2b. Fill in this column with the information you have from the question. Add a total row for the total number of members and fill it in if we know it.
2c. If the question is asking for one of these numbers, mark it with "X", as this will be the number we build the table around to solve for.

In this question, you don't need step 3, but I'll include it for completeness.
3a. Make a third column for the number of memberships including overlaps (e.g., in this case, every food that is liked by every individual).
Note: This number will be greater than the total number of people because by definition some people are members of more than one group (e.g., like more than one food).

3b. Fill in this column with any information we know from the question. If the question is asking for one of these numbers, mark it with "X", as this will be the number we build the table around to solve for.
Note: We may need to sum the total memberships to find the value for the total row here (e.g., 48 like Pizza + 45 like Hoagies + 58 like tacos).

4. Any empty spaces, fill them in by creating expressions using the numbers around them and X. Solve for X.

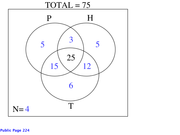
So my summary of Steps 1, 2, and 4 to solve this is in this table:


So the answer is D, as there are 20 people who like either 0 or 1 food.

Just FYI, if the question required it, possible values for Column 3 (Overlaps) could have been:
(X * 0) + Y
60 = 2 foods * 30 members
75 = 3 foods * 25 members
---
151


Thank you Charles! And thank you again, and everyone who helped organise, for making it happen. Trying to help spread the word was the least I could do. I did delay my exam date though, trying to recover the sleep - I am sure you will understand better than most!

75 - (28+37+40) + 25*2 = 20

@empowergmatrich, Can this question be solved using tic-tac-toe? If yes please let me know how.

When you have 3 sets, it's not really possible to set up a grid like other overlapping set questions. The closest thing would be the GMAT Ninja technique I shared above. You can also go with a Venn diagram, but in my opinion that's more complicated and likely to lead to errors (but maybe that would work for your brain better than mine).

Posted from my mobile device

Here is how i tackled this one, will write my thought process in points:

1. We know the whole universe = 75 people, we know that people who like all three =25

3. Simplifying the question stem -- 75 - (people who like ONLY 2 + people who like ONLY 3) = (People who like ONLY 1 + NONE)

4. Recognize that when we are given an overlap of two sets (P&H, H&T ,T&P), we just have to subtract the number of people who like all 3 from each of the terms to get the number of people who like ONLY 2.
5. So, people who like only 2 , i.e P&H, H&T ,T&P = (28-25), (40-25), (37-25) --- > 3 + 12 + 15 = 30
6. Now we know all the terms on one side of the simplified question stem;
75 - (30 +25) = (People who like ONLY 1 + NONE)
20= (People who like ONLY 1 + NONE)

Not sure if this thought process is right or not though Bunuel - do you mind confirming if there are any loop holes in this? Thank you!