'Distance/Speed/Time' Word Problems Made EasyThis post is a part of [
GMAT MATH BOOK]
created by: sriharimurthyedited by: bb,
walker,
Bunuel--------------------------------------------------------
NOTE: In case you are not familiar with translating word problems into equations please go through this post first :
https://gmatclub.com/forum/word-problems ... 87346.htmlWhat is a ‘D/S/T’ Word Problem?- Usually involve something/someone moving at a constant or average speed.
- Out of the three quantities (speed/distance/time), we are required to find one.
- Information regarding the other two will be provided in the question stem.
The ‘D/S/T’ Formula: Distance = Speed x TimeI’m sure most of you are already familiar with the above formula (or some variant of it). But how many of you truly understand what it signifies?
When you see a ‘D/S/T’ question, do you blindly start plugging values into the formula without really understanding the logic behind it? If then answer to that question is yes, then you would probably have noticed that your accuracy isn’t quite where you’d want it to be.
My advice here, as usual, is to make sure you
understand the concept behind the formula rather than just using it blindly.
So what’s the concept? Lets find out!
- The Distance = Speed x Time formula is just a way of saying that the distance you travel depends on the speed you go for any length of time.
If you travel at 50 mph for one hour, then you would have traveled 50 miles. If you travel for 2 hours at that speed, you would have traveled 100 miles. 3 hours would be 150 miles, etc.
If you were to double the speed, then you would have traveled 100 miles in the first hour and 200 miles at the end of the second hour.
- We can figure out any one of the components by knowing the other two.
For example, if you have to travel a distance of 100 miles, but can only go at a speed of 50 mph, then you know that it will take you 2 hours to get there. Similarly, if a friend visits you from 100 miles away and tells you that it took him 4 hours to reach, you will know that he AVERAGED 25 mph. Right?
- All calculations depend on AVERAGE SPEED.
Supposing your friend told you that he was stuck in traffic along the way and that he traveled at 50 mph whenever he could move. Therefore, although practically he never really traveled at 25 mph, you can see how the standstills due to traffic caused his average to reduce. Now, if you think about it, from the information given, you can actually tell how long he was driving and how long he was stuck due to traffic (assuming; what is false but what they never worry about in these problems; that he was either traveling at 50 mph or 0 mph). If he was traveling constantly at 50 mph, he should have reached in 2 hours. However, since he took 4 hours, he must have spent the other 2 hours stuck in traffic!
- Now lets see how we can represent this using the formula.
We know that the total distance is 100 miles and that the total time is 4 hours. BUT, his rates were different AND they were different at different times. However, can you see that no matter how many different rates he drove for various different time periods, his TOTAL distance depended simply on the SUM of each of the different distances he drove during each time period?
E.g., if you drive a half hour at 60 mph, you will cover 30 miles. Then if you speed up to 80 mph for another half hour, you will cover 40 miles, and then if you slow down to 30 mph, you will only cover 15 miles in the next half hour. But if you drove like this, you would have covered a total of 85 miles (30 + 40 + 15). It is fairly easy to see this looking at it this way, but it is more difficult to see it if we scramble it up and leave out one of the amounts and you have to figure it out going "backwards". That is what word problems do.
Further, what makes them difficult is that the components they give you, or ask you to find can involve variable distances, variable times, variable speeds, or any two or three of these. How you "reassemble" all this in order to use the d = s*t formula takes some reflection that is "outside" of the formula itself. You have to think about how to use the formula.
So the trick is to be able to understand EXACTLY what they are giving you and EXACTLY what it is that is missing, but you do that from thinking, not from the formula, because the formula only works for the COMPONENTS of any trip where you are going an average speed for a certain amount of time. ONCE the conditions deal with different speeds or different times, you have to look at each of those components and how they go together. And that can be very difficult if you are not methodical in how you think about the components and how they go together. The formula doesn't tell you which components you need to look at and how they go together. For that, you need to think, and the thinking is not always as easy or straightforward as it seems like it ought to be.
In the case of your friend above, if we call the time he spent driving 50 mph, T1; then the time he spent standing still is (4 - T1) hours, since the whole trip took 4 hours. So we have 100 miles = (50 mph x T1) + (0 mph x [4 - T1])
which is equivalent then to: 100 miles = 50 mph x T1
So, T1 will equal 2 hours. And, since the time he spent going zero is (4 - 2), it also turns out to be 2 hours.
- Sometimes the right answers will seem counter-intuitive, so it is really important to think about the components methodically and systematically.
There is a famous trick problem: To qualify for a race, you need to average 60 mph driving two laps around a 1 mile long track. You have some sort of engine difficulty the first lap so that you only average 30 mph during that lap; how fast do you have to drive the second lap to average 60 for both of them?
I will go through THIS problem with you because, since it is SO tricky, it will illustrate a way of looking at almost all the kinds of things you have to think about when working any of these kinds of problems FOR THE FIRST TIME (i.e., before you can do them mechanically because you recognize the TYPE of problem it is). Intuitively it would seem you need to drive 90, but this turns out to be wrong for reasons I will give in a minute.
The answer is that NO MATTER HOW FAST you do the second lap, you can't make it. And this SEEMS really odd and that it can't possibly be right, but it is. The reason is that in order to average at least 60 mph over two one-mile laps, since 60 mph is one mile per minute, you will need to do the whole two miles in two minutes or less. But if you drove the first mile at only 30, you used up the whole two minutes just doing IT. So you have run out of time to qualify.
To see this with the d = s*t formula, you need to look at the overall trip and break it into components, and that is the hardest part of doing this (these) problem(s), because (often) the components are difficult to figure out, and because it is hard to see which ones you need to put together in which way.
In the next section we will learn how to do just that.
Resolving the Components- When you first start out with these problems, the best way to approach them is by organizing the data in a tabular form.
Use a separate column each for distance, speed and time and a separate row for the different components involved (2 parts of a journey, different moving objects, etc.). The last row should represent total distance, total time and average speed for these values (although there might be no need to calculate these values if the question does not require them).
Attachment:
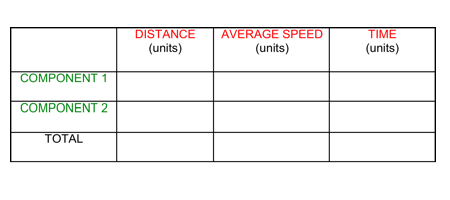 General.png [ 17.44 KiB | Viewed 942646 times ]
General.png [ 17.44 KiB | Viewed 942646 times ]
- Assign a variable for any unknown quantity.
If there is more than one unknown quantity, do not blindly assign another variable to it. Look for ways in which you can express that quantity in terms of the quantities already present. Assign another variable to it only if this is not possible.
- In each row, the quantities of distance, speed and time will always satisfy d = s*t.
- The distance and time column can be added to give you the values of total distance and total time but you CANNOT add the speeds.
Think about it: If you drive 20 mph on one street, and 40 mph on another street, does that mean you averaged 60 mph?
- Once the table is ready, form the equations and solve for what has been asked!
Warning: Make sure that the units for time and distance agree with the units for the rate. For instance, if they give you a rate of feet per second, then your time must be in seconds and your distance must be in feet. Sometimes they try to trick you by using the wrong units, and you have to catch this and convert to the correct units.
A Few More Points to Note- Motion in Same Direction (Overtaking): The first thing that should strike you here is that at the time of overtaking, the distances traveled by both will be the same.
- Motion in Opposite Direction (Meeting): The first thing that should strike you here is that if they start at the same time (which they usually do), then at the point at which they meet, the time will be the same. In addition, the total distance traveled by the two objects under consideration will be equal to the sum of their individual distances traveled.
- Round Trip: The key thing here is that the distance going and coming back is the same.
Now that we know the concept in theory, let us see how it works practically, with the help of a few examples.Note for tables : All values in
black have been given in the question stem. All values in
blue have been calculated.
Example 1.To qualify for a race, you need to average 60 mph driving two laps around a 1-mile long track. You have some sort of engine difficulty the first lap so that you only average 30 mph during that lap; how fast do you have to drive the second lap to average 60 for both of them?
Solution:Let us first start with a problem that has already been introduced. You will see that by clearly listing out the given data in tabular form, we eliminate any scope for confusion.
Attachment:
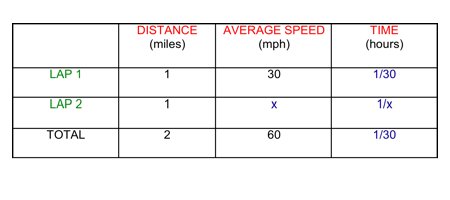 Eg.1.png [ 17.55 KiB | Viewed 941999 times ]
Eg.1.png [ 17.55 KiB | Viewed 941999 times ]
In the first row, we are given the distance and the speed. Thus it is possible to calculate the time.
Time(1) = Distance(1)/Speed(1) = 1/30In the second row, we are given just the distance. Since we have to calculate speed, let us give it a variable 'x'. Now, by using the 'D/S/T' relationship, time can also be expressed in terms of 'x'.
Time(2) = Distance(2)/Speed(2) = 1/xIn the third row, we know that the total distance is 2 miles (by taking the sum of the distances in row 1 and 2) and that the average speed should be 60 mph. Thus we can calculate the total time that the two laps should take.
Time(3) = Distance(3)/Speed(3) = 2/60 = 1/30Now, we know that the total time should be the sum of the times in row 1 and 2. Thus we can form the following equation :
Time(3) = Time(1) + Time(2) ---> 1/30 = 1/30 + 1/xFrom this, it becomes clear that '1/x' must be 0.
Since 'x' is the reciprocal of 0, which does not exist, there can be no speed for which the average can be made up in the second lap.Example 2.An executive drove from home at an average speed of 30 mph to an airport where a helicopter was waiting. The executive boarded the helicopter and flew to the corporate offices at an average speed of 60 mph. The entire distance was 150 miles; the entire trip took three hours. Find the distance from the airport to the corporate offices.
Solution:Since we have been asked to find the distance from the airport to the corporate office (that is the distance he spent flying),
let us assign that specific value as 'x'. Thus, the distance he spent driving will be '150 - x'Now,
in the first row, we have the distance in terms of 'x' and we have been given the speed. Thus we can calculate the time he spent driving in terms of 'x'.
Time(1) = Distance(1)/Speed(1) = (150 - x)/30Similarly,
in the second row, we again have the distance in terms of 'x' and we have been given the speed. Thus we can calculate the time he spent flying in terms of 'x'.
Time(2) = Distance(2)/Speed(2) = x/60Now, notice that we have both the times in terms of 'x'. Also, we know the total time for the trip. Thus, summing the individual times spent driving and flying and equating it to the total time, we can solve for 'x'.
Time(1) + Time(2) = Time(3) --> (150 - x)/30 + x/60 = 3 --> x = 120 milesAnswer : 120 milesNote: In this problem, we did not calculate average speed for row 3 since we did not need it. Remember not to waste time in useless calculations!
Example 3.A passenger train leaves the train depot 2 hours after a freight train left the same depot. The freight train is traveling 20 mph slower than the passenger train. Find the speed of the passenger train, if it overtakes the freight train in three hours.
Solution:Since this is an 'overtaking' problem, the first thing that should strike us is that the distance traveled by both trains is the same at the time of overtaking.
Next we see that we have been asked to find the speed of the passenger train at the time of overtaking.
So let us represent it by 'x'. Also, we are given that the freight train is 20 mph slower than the passenger train.
Hence its speed in terms of 'x' can be written as 'x - 20'.Moving on to the time, we are told that it has taken the passenger train 3 hours to reach the freight train. This means that the passenger train has been traveling for 3 hours.
We are also given that the passenger train left 2 hours after the freight train. This means that the freight train has been traveling for 3 + 2 = 5 hours.
Now that we have all the data in place, we need to form an equation that will help us solve for 'x'. Since we know that the distances are equal, let us see how we can use this to our advantage.
From the first row, we can form the following equation :
Distance(1) = Speed(1) * Time(1) = x*3From the second row, we can form the following equation :
Distance(2) = Speed(2) * Time(2) = (x - 20)*5Now, equating the distances because they are equal we get the following equation :
3*x = 5*(x - 20) --> x = 50 mph.Answer : 50 mph.Example 4.Two cyclists start at the same time from opposite ends of a course that is 45 miles long. One cyclist is riding at 14 mph and the second cyclist is riding at 16 mph. How long after they begin will they meet?
Solution:Since this is a 'meeting' problem, there are two things that should strike you. First, since they are starting at the same time, when they meet, the time for which both will have been cycling will be the same. Second, the total distance traveled by the will be equal to the sum of their individual distances.
Since we are asked to find the time, let us assign it as a variable 't'. (which is same for both cyclists)
In the first row, we know the speed and we have the time in terms of 't'. Thus we can get the following equation :
Distance(1) = Speed(1) * Time(1) = 14*tIn the second row, we know the speed and again we have the time in terms of 't'. Thus we can get the following equation :
Distance(2) = Speed(2) * Time(2) = 16*tNow we know that the total distance traveled is 45 miles and it is equal to the sum of the two distances. Thus we get the following equation to solve for 't' :
Distance(3) = Distance(1) + Distance(2) --> 45 = 14*t + 16*t --> t = 1.5 hoursAnswer : 1.5 hours.Example 5.A boat travels for three hours with a current of 3 mph and then returns the same distance against the current in four hours. What is the boat's speed in calm water?
Solution:Since this is a question on round trip, the first thing that should strike us is that the distance going and coming back will be the same.
Now, we are required to find out the boats speed in calm water. So let us assume it to be 'b'. Now if speed of the current is 3 mph, then the speed of the boat while going downstream and upstream will be 'b + 3' and 'b - 3' respectively.
In the first row, we have the speed of the boat in terms of 'b' and we are given the time. Thus we can get the following equation :
Distance(1) = Speed(1) * Time(1) = (b + 3)*3In the second row, we again have the speed in terms of 'b' and we are given the time. Thus we can get the following equation :
Distance(2) = Speed(2) * Time(2) = (b - 3)*4Since the two distances are equal, we can equate them and solve for 'b'.
Distance(1) = Distance(2) --> (b + 3)*3 = (b - 3)*4 --> b = 21 mph.Answer : 21 mph.__________________________________________________________
For More On Distance/Rate Problems Check Under The Spoiler
Show SpoilerFor More On Distance/Rate Problems Check Under The Spoiler
