How to Solve: Statistics
Attached pdf of this Article as SPOILER at the top! Happy learning! 
Hi All,
I have recently uploaded a video on YouTube to discuss Statistics Basics in Detail:
Following is covered in the video
• Mean and Properties of Mean
• Median and Properties of Median
• Range and Properties of Range
• Mode
• Weighted Mean
• Variance and Properties of Variance
• Standard Deviation and Properties of SDTheoryMean /Average / Arithmetic Mean• Mean is the average of the all the numbers in the set.
• Mean = \(\frac{Sum Of All The Numbers In The Set }{ Total Number Of Numbers In The Set}\)
Suppose the set is {1,2,3,4,5}
Then, Mean = \(\frac{(1+2+3+4+5)}{5} \)=\(\frac{ 15 }{ 5} \)= 3
Properties of Mean1. If all the numbers in the set are increased/decreased by the same number(k) then the mean also gets increased/decreased by the same number(k)
Suppose the set is {a,b,c,d,e}
then the Mean = \(\frac{(a+b+c+d+e)}{5}\)
Now, lets increase all the numbers by k. So, the new set is {a+k,b+k,c+k,d+k,e+k)
New Mean = \(\frac{(a+k +b+k +c+k +d+k + e+k)}{5}\)
= \(\frac{(a+b+c+d+e + 5k)}{5}\) = \(\frac{(a+b+c+d+e)}{5}\) + k = Old Mean + k
2. If all the numbers in the set are multiplied/divided by the same number(k) then the mean also gets multiplied/divided by the same number(k)
Proof same as above. In this case if we multiple all the numbers by k then
New Mean = k* (Old Mean)
SUGGESTION: Don't try remembering the points 1 and 2 above. It does not take much time to calculate them!
Median• Median is the middle value of the set.
• In case of even number of numbers in the set: Median is the mean of the two middle numbers (after the numbers are arranged in the increasing / decreasing order)
Example: If the set is {5,1,4,6,3,2} then we will arrange the set as {1,2,3,4,5,6} and median will be mean of middle two terms. Middle two terms in this case are 3 and 4 so Median = (3+4)/2 = 3.5
• In case of odd number of numbers in the set: Median is the middle number (after the numbers are arranged in increasing/ decreasing order )
Example: If the set is {4,5,3,1,2} then we will arrange the set as {1,2,3,4,5} and the median will be the middle number which is 3
Properties of Median1. If all the numbers in the set are increased/decreased by the same number(k) then the median also gets increased/decreased by the same number(k)
Proof same as for mean.
2. If all the numbers in the set are multiplied/divided by the same number(k) then the median also gets multiplied/divided by the same number(k)
Proof same as for mean.
3. In Case of evenly spaced set
Mean = Median = Middle term (if the number of terms is odd)
= Mean of middle terms (if the number of terms is even)
4. In case of consecutive integers: IF the number of integers is even then then the Mean = Median ≠ Integer
Suppose the set is {1,2,3,4,5,6}
then Mean = Median = 3.5
SUGGESTION: Don't try remembering the points 1 and 2 above. It does not take much time to calculate them!
Range• Range of a set is the difference between the highest and lowest value of the set.
Example: Suppose the set is {-1,2,3,6,8} then the range will be
8 -(-1) = 9
Properties of Range1. If all the numbers in the set are increased/decreased by the same number(k) then the range DOES NOT CHANGE!
Suppose the set is {a,b,c} (in increasing order)
Range = c-a
Now, lets increase all the numbers by k then the set will become {a+k, b+k, c+k}
New range = c+k -(a+k) = c-a = Old range
2. If all the numbers in the set are multiplied/divided by the same number(k) then the range also gets multiplied/divided by the same number(k)
Proof similar to that for mean.
Mode• Mode is the number which has occurred the maximum number of times in the set.
Suppose the set is {1,1,2,2,3,3,3,3,4,5}
then the mode is 3, as 3 has occurred the maximum number of times in the set.
Weighted Average• Weighted Average = \(\frac{((Weight_1∗Value_1) + (Weight_2∗Value_2)…+ (Weight_N∗Value_N))}{(Sum Of Weight)}\)
Q1. If an employee’s performance consists of 20% of component A, 30% of component B and 50% of component C and if he receives 10 in A, 20 in B and 10 in C, then find the overall performance of the employee
Ans 13 (Check
Video for solution)
Variance• Variance, V = Mean of (Square of difference of each number from the mean)
V = \(\frac{Sum of (Squares Of Difference Of Each Number From Mean) }{Total Number Of Numbers }\)
Q1 Find the Variance of the set { 1, 2, 3, 4, 5 }
Sol: Mean of this set is 3
Variance, V = \(\frac{((3-1)^2 + (3-2)^2 + (3-3)^2 + (3-4)^2 + (3-5)^2)}{ 5 }\)
= \(\frac{(4+1+0+1+4)}{5}\) = 2
Variance Properties1. If all the numbers in a set are increased/ decreased by the same number(k) then the variance DOES NOT change
Check
Video For Explanation
2. If all the numbers in a set are multiplied/ divided by the same number(k) then the variance gets multiplied/divided by the square of the number (\(k^2\))
Check
Video For Explanation
Standard Deviation(SD)• SD is an indication of how spread the numbers are as compared to the Mean
• SD is equal to the Root Mean Square(RMS) of the distance of the values from the mean
• Standard Deviation =\( \sqrt{Variance}\), SD = \(\sqrt{V}\)
Q1 Find the SD of the set { 1, 2, 3, 4, 5 }
Sol: V = 2 (calculated above)
SD = \(\sqrt{V}\) = \(\sqrt{2}\)
SD Properties1. If all the numbers in the set are increased/decreased by the same number(k) then the Standard Deviation DOES NOT CHANGE!
(This happens because the mean also gets increased/decreased by the same number and the Variance or Standard Deviation are calculated by subtracting all the numbers by the mean and taking square of them and taking their average. )
2. If all the numbers in the set are multiplied/divided by the same number(k) then the Standard Deviation also gets multiplied/divided by the modulus of that number. ( |k| ).
Zero SD• SD of a 1 element set
Check
Video For Explanation
• SD of a set with all numbers equal
Check
Video For Explanation
Recap of PropertiesAttachment:
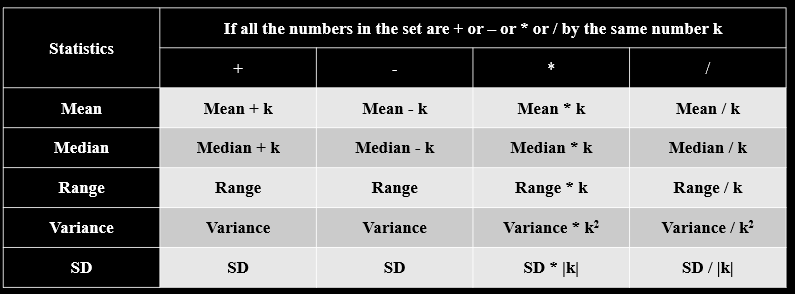 Relationships.png [ 94.25 KiB | Viewed 2254 times ]
Relationships.png [ 94.25 KiB | Viewed 2254 times ]
Arithmetic Sequence is the sequence in which each number differs from its previous by a constant value (d)
Arithmetic sequence is generally denoted as
a , a+d , a+2d+,..., a+(n-1)d
where, a is the first term of the sequence.
d is the common difference
n is the number of terms in the sequence.
Tn is the nth term of the sequence.
Tn = a + (n-1)d
A.M. = Arithmetic mean of the sequence = Mean of First term and last term = (a + a+(n-1)d)/2
= (2a+ (n-1)d)/2
Sum of all the terms of the sequence = A.M. * n = (n/2) * (2a + (n-1)d)
Problems:1. If the mean of numbers 28, x, 42, 78 and 104 is 62, then what is the mean of 128, 255, 511, 1023 and x?
A. 395
B. 275
C. 355
D. 415
E. 365
Solution:
the mean of numbers 28, x, 42, 78 and 104 is 62
=> (28+x+42+78+104)/5 = 62
=> x = 58
the mean of 128, 255, 511, 1023 and x = mean of 128, 255, 511, 1023 and 58
= (128+255+511+1023+58)/5 = 1975/5 = 395
So, Answer will be A
link to the problem:https://gmatclub.com/forum/if-the-mean-of-numbers-28-x-42-78-and-104-is-62-then-135288.html2. Set S consists of 5 values, not necessarily in ascending order: {4, 8, 12, 16, x}. For how many values of x does the mean of set S equal the median of set S?
(A) Zero
(B) One
(C) Two
(D) Three
(E) More than three
Solution:
we need to first decide where will we position x
suppose we keep x at the center then the set in ascending order will be
{4,8,x,12,16}
now the median = x so the mean also has to be x
=> (4+8+x+12+16)/5 = x
=> x= 10
which is possible. So, x=10 is one such value
Now lets put x at the left of 8 so the median will be 8 now. So even the mean has to be 8
=> (4+8+x+12+16)/5 = 8
=> x= 0
Which is possible. So, x=0 is one such value
Now lets put x at the right of 12 so the median will be 12 now. So even the mean has to be 12
=> (4+8+x+12+16)/5 = 12
=> x= 20
Which is possible. So, x=20 is one such value
So, there are three such values for which mean = median.
So, answer will be D
link to the problem:https://gmatclub.com/forum/set-s-consists-of-5-values-not-necessarily-in-ascending-144018.html3.
https://gmatclub.com/forum/set-a-3-3-3-4-5-5-5-has-a-standard-deviation-of-138266.htmlLooking for a Quant Tutor?
Check out my post for the same
https://gmatclub.com/forum/starting-gmat ... 35537.htmlHope it helps!
Good Luck!

