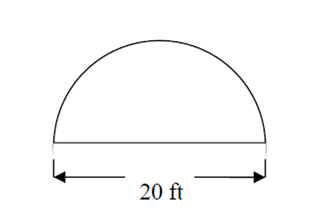
The figure above shows the dimensions of a semicircular cross section

radius =10
Say AOB is base of the semi circle.
points X and Y are on edges of the traffic lane (XY=12)
OX=6

Find the height perpendicular to X and touches the semi circle.
= sqrt (6^2+10^2) =8

we need 1/2 ft clearance.

so height must be (8-1/2) =71/2 ft


B.

Interesting question!

I'll try to explain as best as I can without drawing a diagram - sadly, I don't have the computer resources to do so (or at least I don't know how!).

Let's label the midpoint of the circle O. Since the base of the semi-circle is 20, we know that the diameter is 20 and, accordingly, the radius is 10.

We also know that the traffic lane is 12 feet long and there's an equal amount of space on either side, so the traffic lane extends 6 feet on either side of O. Let's call the leftmost point on the base of the traffic lane A. So, the distance OA is 6.

Now draw a line straight up from A to the top of the tunnel. Let's label the point at which the line intersects the circle B. The answer to the question will, therefore, be the height AB - .5 feet (we need to leave .5 feet of clearance).

Here's the key to solving the question: if we draw a line from O to B, that line is a radius of the circle and, therefore, has length 10. We now have right triangle OAB (the right angle is at point A), with leg OA=6 and hypotenuse OB=10. We can now solve for leg AB=8 (either by applying the pythagorean theorum or by applying the 3/4/5 special right triangle ratio).

Finally: AB=8, so the correct answer is 8 - .5 = 7.5... choose (B)!

* * *

From a strategic guessing point of view, as soon as we realize that the height of the tunnel is 10 in the middle, we should quickly eliminate (D) and (E) as too big; worse case you have a 1/3 shot at picking up the points.

Amazing skovinsky,
I cant understand how i was not able to crack this. This must be very basic question...I did everything just last triangle thing i was not able to think. +1 kudo for you....

Heh - this is definitely not a very basic question.

Here are a few takeaways for future questions:

1) whenever you see a multiple figure question involving circles, think about how the radius and/or diameter of the circle relate to the other shape. Since the radius is the base unit of a circle, it's often the key to solving the problem.

2) when triangles are involved, consider the common special right triangles (\(3x:4x:5x, 5x:12x:13x, x:x:x\sqrt{2}, x:x\sqrt{3}:2x\)) and their application to the question.

3) when stuck with the math, use logic and common sense to at least eliminate some choices to increase your odds.

Hi Guys!

I was thinking about extreme cases, such a truck with cylidrical tank. In this case you would not take into account the width of the lane. Hence the maximum height of the truck would be R - 1/2 = 10-1/2 = 9 1/2 (where R is the radius of the semicircular cross).



Unfortuantely, this answer is wrong, but I still think that it is valid. Can somebody explain why I'm wrong?
THX!

Hi, late response but it might be helpful to someone else. The reason is because any truck can only have a maximum width = width of the lane, that's what the problem is saying. that means, there are parts of the truck, end points on two sides that can only rise to the height limited by the ceiling of the tunnel at those end points, which is the distance we are trying to find out = 8. hope it's clear.

I have question here, if in the above question they sid that If vehicles must clear the top of the

tunnel by at least 1/2 foot when they are inside the traffic lane. So, they ask about the max

height of the vehicles that allowed to cross the tunnel.We have the radius = 10. So max height of

the vehicle that are allowed to use the tunnel is 10- 0.5 = 9.5 what is the wrong here?

The truck has a width. The entire truck must pass through the semi circular tunnel. I did not get the image I had in mind but look here:


Now imagine that the tunnel is semi circular. If the truck has height of 9.5, its edges will not pass through the sides. Its height needs to be lesser. Since the traffic lane is 12 feet wide and vehicles must stay inside traffic lane, the maximum width of the vehicle will be 12 feet and that will set the limit on the maximum height allowed.

Alt Solution

please refer image

Hi I am not clear as to why is OB=6 ? (How did we arrive at 12/2?)

The single traffic lane is 12 feet wide and is equidistant from the sides of the tunnel. OB is half of the lane, which is 12 feet.

Does anyone else think the question is too ambiguous and subject to interpretation?
The question states nothing about the width of the vehicle passing through. Ofcourse if we just subtract 10-0.5 =9.5 feet, the question stem about traffic lane being equidistant from the sides seems unnecessary.
Now I understand that we have to take the width into consideration as well but why do we have to assume the the top of the traffic lane to be horizontally flat and not curved?

not OG question hence ambiguity. think of it differently. the point is to think a bit beyond what q simply asks. If you can tell that it's ambiguous from the beginning then that's all you need to know. If you couldn't tell it's ambiguous, then don't jump to conclusions either way.

Hello Bunuel sir,
Can you please explain what is wrong in my understanding?
Here, the width of lane is 12 feet. So, it is the maximum width that a vehicle can have. It can be less than 12 feet. But why we assume that the width of vehicle is only 12 feet.

If the vehicle width is 10 feet, then the height will be : 10^2 - 5^2 = h^2 or, h = \sqrt{75}.
So, the max height is = \sqrt{75} - 0.5

If the vehicle width is 8 feet, then the height will be : 10^2 - 4^2 = h^2 or, h = \sqrt{84}.
So, the max height is = \sqrt{84} - 0.5

and so on.

So why the official answer is 7.5.

Thank you.

When we construct the requisite diagram we get a 6-8-10 triangle
6 is refering to the single lane trafiic
10 is the radius oe the end point of the traffic
8 is the height and the answer should 8-0.5

Therefore IMO B

KarishmaB
Thank you for your help explanation. Are there other problems in this nature that I can practice that you have come across? I really struggled with this one!

STRATEGY:
We will translate the question from English to Math as we read it. This way, we’ll clearly get all that is given to us and that we need to find.

TIP: Simultaneous translation will help you understand a question in thorough detail and leave no scope for confusion as you progress through the solution.

Let’s go!


GIVEN – Understand the given information – TRANSLATE and VISUALIZE:

• A one-way tunnel has a semicircular cross section.
  
  • The given figure shows the diameter of the tunnel as 20 ft. So, the radius of the semicircular tunnel = 20/2 = 10 ft.
• A 12 ft wide traffic lane is equidistant from the sides of the tunnel.
  
  • Say, the traffic lane is at an equal distance of x ft from both sides of the tunnel.
  • Then x + 12 + x = 20.
  • This implies x = 4.
    
• Any vehicle inside the lane MUST have a gap of at least ½ foot between the vehicle top and the ceiling of the tunnel.
  
  • This distance is calculated for every point on the vehicle top to the point on the tunnel’s ceiling directly above.

I know this last bit seems confusing. Let’s VISUALIZE this through some examples:
In the figure below, rectangle ABCD represents a vehicle passing through the tunnel. Suppose ‘h’ is the height of the vehicle (that is, AB = CD = h).


Required distance between vehicle and tunnel’s ceiling:

• At point A: Distance between point A on the top of the vehicle and point P on the tunnel’s ceiling directly above A = AP
• At point D: Distance between point D on the top of the vehicle and the point Q on the tunnel’s ceiling directly above D = DQ
• At point E (say, this is the midpoint of AD): Distance between point E on the top of the vehicle and the point R on the tunnel’s ceiling directly above E = ER

Now, per the question, each of these distances needs to be at least ½ foot.

OBSERVE: AP < ER and similarly, DQ < ER.
In fact, AP = DQ is the minimum distance between the vehicle and the tunnel. This is where the vehicle top will be the closest to the tunnel’s ceiling.
So, if AP or DQ is >= ½ foot, then we can safely say that the vehicle’s top is always at least ½ foot away from the tunnel’s ceiling. ----(I)


TO FIND – Understand the question - TRANSLATE:

• Limit on the height of the vehicles that are allowed inside the tunnel.
  
  • That is, the maximum possible value of ‘h’.

SOLUTION:
Now that we understand the question, finding the final answer will be easy. Let’s just bring back the figure we drew.

Visualize:


As explained in (I), the gap between the vehicle’s top and the tunnel’s ceiling is minimum at the extreme points A and D on the top. So, if this minimum gap (AP) is at least ½ foot, then we’re done.

Observe that AP = BP – h. Then, AP ≥ ½ implies that:

• BP – h ≥ ½.
• h ≤ BP – ½ ----(II)

So, we are left with finding BP to get the required value of h.


Finding the value of BP:
Let us re-draw the above figure with only that is needed. Also, suppose the center of the tunnel/traffic lane is W, as shown.

Now, since triangle WBP is a right triangle, we just need the lengths of BW and WP to find BP.

• Since the traffic lane is given to be 12 ft wide, BC = 12.
  
  • And since W is the center of the lane, BW = \(\frac{12}{2}\)= 6 ft. --- (III)
• Also, WP is nothing but the radius of the semicircular tunnel.
  
  • So, WP = radius = 10 ft. --- (IV)

Finally, by applying the Pythagoras’ theorem on △WBP, we have:

\(WP^2 = BP^2 + WB^2\)
\(10^2 = BP^2 + 6^2\) (Using III and IV)
\(BP^2 \)= 100 – 36 = 64

So, BP = 8 (As height cannot be negative) ----(V)


Finding the value of ‘h’:
Using (II) and (V), we get:

• h ≤ 8 – ½
• h ≤ 7 ½

So, the maximum possible value of h is 7 ½ ft. That is, the maximum possible height of any vehicle that can pass through the tunnel while meeting the conditions given is 7 ½ ft.


Correct Answer: Option B



Best,
Aditi Gupta
Quant expert, e-GMAT