Events & Promotions
|
|
GMAT Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
Mar 2511:00 AM PDT
-12:00 PM PDT
Dreaming about studying abroad but worried about finances? In this video, we provide overview of HDFC Credila's education loan offerings and insights into securing an education loan effortlessly.
Mar 1910:00 AM EDT
-11:59 PM EDT
Get a massive $250 off the TTP OnDemand GMAT course by using the coupon code FLASH25 at checkout. If you prefer learning through engaging video lessons, TTP OnDemand GMAT is exactly what you need.- Mar 24
08:30 AM PDT
-09:30 AM PDT
Join 99th percentile Manhattan Prep Instructor, Ryan Starr, for the third session in our three-part series on how to maximize your study effectiveness. The new GMAT tests comprehension, analysis, and logic, and more. - Mar 26
10:00 AM PDT
-11:00 AM PDT
Confused about how to get the most from your GMAT mock tests? In this video, learn the perfect strategy to analyze GMAT mocks effectively and boost your score. We break down the common mistakes GMAT aspirants make when using mock tests 
Mar 2610:00 AM EDT
-11:59 PM EDT
The Target Test Prep GMAT Flash Sale is LIVE! Get 25% off our game-changing course and save up to $400 today! Use code FLASH25 at checkout. This limited-time deal won’t last long, so grab your discount now!- Mar 27
09:30 AM PDT
-10:30 AM PDT
Preparing for the GMAT? Avoid these 5 major mistakes that can lower your score and hurt your MBA dreams. In this expert panel discussion, 4 top GMAT coaches - GMAT Ninja, Jeff Miller from TTP, Rida Shafiq from eGMAT, and Chris Gentry from Manhattan Prep - Mar 27
10:00 AM PDT
-11:00 AM PDT
What happens after getting an MBA? Is it worth the investment? To find out, I interviewed 10 GMAT Club professionals who have completed their MBAs and built careers in consulting, finance, tech, and entrepreneurship. Their answers might surprise you! 
Mar 2911:30 AM EDT
-12:30 PM EDT
Learn how Keshav, a Chartered Accountant, scored an impressive 705 on GMAT in just 30 days with GMATWhiz's expert guidance. In this video, he shares preparation tips and strategies that worked for him, including the mock, time management, and more.
Mar 3011:00 AM IST
-01:00 PM IST
Attend this session to evaluate your current skill level, learn process skills, and solve through tough Arithmetic questions.
01 Dec 2023, 09:32
Kudos
Bookmarks
C
Be sure to select an answer first to save it in the Error Log before revealing the correct answer (OA)!
Difficulty:
35%
(medium)
Question Stats:
78% (02:27) correct
22%
(02:34)
wrong
based on 459
sessions
History
Date
Time
Result
Not Attempted Yet
The number of hours required for a certain company to produce 1 unit of products X, Y, and Z, respectively, are shown in the table. Products X and Y can be produced simultaneously, and so can products X and Z; but products Y and Z cannot be produced simultaneously. What is the least number of hours required to produce a total of 10 units of product X, 5 units of product Y, and 7 units of product Z?
A. 35
B. 45
C. 55
D. 65
E. 85
Attachment:
2023-12-01_19-54-12.png [ 11.2 KiB | Viewed 13265 times ]
Attachment:
2023-12-01_19-55-24.png [ 74.91 KiB | Viewed 9913 times ]
01 Dec 2023, 09:38
Kudos
Bookmarks
guddo
The number of hours required for a certain company to produce 1 unit of products X, Y, and Z, respectively, are shown in the table. Products X and Y can be produced simultaneously, and so can products X and Z; but products Y and Z cannot be produced simultaneously. What is the least number of hours required to produce a total of 10 units of product X, 5 units of product Y, and 7 units of product Z?
A. 35
B. 45
C. 55
D. 65
E. 85
Attachment:
2023-12-01_19-54-12.png
Attachment:
2023-12-01_19-55-24.png
Since Y and Z cannot be produced simultaneously, let's calculate the amount of time required to produce 5 items of Y and 7 items of Z:
- 5 items of Y would require 5*4 = 20 hours.
- 7 items of Z would require 7*5 = 35 hours.
Since these products cannot be produced simultaneously, producing 5 items of Y and 7 items of Z together would require 20 + 35 = 55 hours.
Now, since producing 10 items of X requires 10*3 = 30 hours, and since item X can be paired either with products Y or Z, we can produce products X during those 55 hours without requiring additional time.
Therefore, only 55 hours are required to produce the required number of items.
Answer: C.
General Discussion
01 Dec 2023, 23:07
Kudos
Bookmarks
guddo
The number of hours required for a certain company to produce 1 unit of products X, Y, and Z, respectively, are shown in the table. Products X and Y can be produced simultaneously, and so can products X and Z; but products Y and Z cannot be produced simultaneously. What is the least number of hours required to produce a total of 10 units of product X, 5 units of product Y, and 7 units of product Z?
A. 35
B. 45
C. 55
D. 65
E. 85
As we require the least number of hours, we need to ensure that the products are produced as simultaneously as possible.
We can divide the production into two groups -
- Group 1: X & Y ⇒ X & Y produces simultaneously
- Group 2: X & Z ⇒ X & Z produces simultaneously
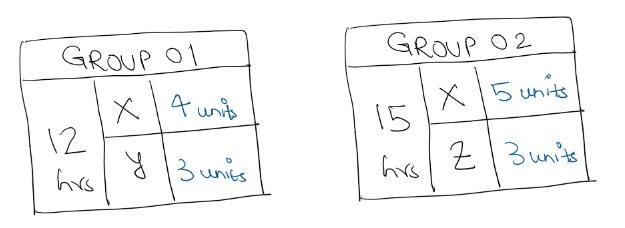
For Group 1, in 12 hours, 4 units of X are produced and 3 units of Y are produced.
For Group 2, in 15 hours, 5 units of X are produced and 3 units of Z are produced.
Attachment:
Screenshot 2023-12-02 111515.png [ 37.38 KiB | Viewed 11921 times ]
Assume that the production of X and Y is simultaneous, hence, in 12 hours we will have 4 units of X and 3 units of Y. After 24 hours, we will have 8 units of X and 6 units of Y. We need only 5 units of Y, the production of X and Y can run simultaneously for 24 - 4 = 20 hours.
In 20 hours we have produced 6 units of X and 5 units of Y.
We still need 4 units of X and 7 units of Z. Now the production of X and Z can start. As fewer units of X is required and the production of X is faster than the production of Z, the time required will depend on the time to produce 7 units of Z.
Time required to produce 7 units of Z = 7 * 5 = 35 hours
Total time required = 35 + 20 = 55 hours
Hence, we can eliminate D and E as they are greater than 55. Option A cannot be true as well, as 7 * 5 = 35. This is possible when the production of X, Y, and Z occurs simultaneously.
Option B, 45, is not possible as well, because the production of 7 units of Z alone takes 35 hours. Hence, if we produce X and Z together and let the production run for 35 hours. We will still need at least 5 * 4 = 20 hours to produce Y alone. Hence, 55 is the least possible hours.
Option C
