liranmaymoni wrote:
At 7:57 am, Flight 501 is at an altitude of 6 miles above the ground and is on a direct approach (i.e., flying in a direct line to the runway) towards The Airport, which is located exactly 8 miles due north of the plane’s current position. Flight 501 is scheduled to land at The Airport at 8:00 am, but, at 7:57 am, the control tower radios the plane and changes the landing location to an airport 15 miles directly due east of The Airport. Assuming a direct approach (and negligible time to shift direction), by how many miles per hour does the pilot have to increase her speed in order to arrive at the new location on time?
A. \(5\sqrt{13} - 10\) miles/hr
B. 100 miles/hr
C. \(100\sqrt{13} - 200\) miles/hr
D. 200 miles/hr
E. \(100\sqrt{13}\) miles/hr
There are 3 right triangles here in different planes. On the ground, we have the two airports 15 miles apart (due east) and the plane is 8 miles to the south of A1 (point G). So this is the 8-15-17 triangle.
Attachment:
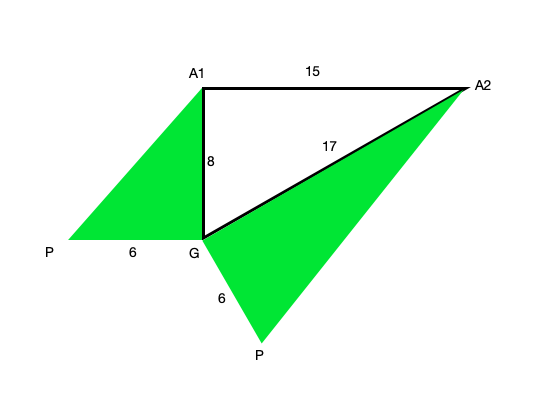 Screenshot 2021-03-02 at 14.54.50.png [ 25.07 KiB | Viewed 9759 times ]
Screenshot 2021-03-02 at 14.54.50.png [ 25.07 KiB | Viewed 9759 times ]
The points P represent the current location of the plane at height 6. The green triangles are in the air with base on the ground. The plane needs to travel from P to A1 or A2 in the two situations.
P to A1 - Distance is 10
Time taken = 3 mins
\(Speed = \frac{10}{3/60} miles/hr = 200 miles/hr\)
P to A2 - Distance is \(\sqrt{17^2 + 6^2} = 5\sqrt{13}\)
Time taken = 3 mins
\(Speed = \frac{5\sqrt{13}}{(3/60)} = 100\sqrt{13}\) miles/hr
\(Increase = (100\sqrt{13} - 200)\) miles/hr
Answer (C)



 95%
(hard)
95%
(hard)
 45%
(03:36)
wrong
45%
(03:36)
wrong  based on 265
sessions
based on 265
sessions



