If a circle passes through points (9,2*sqrt(3))

My algebra as usual makes me cry: I can't get how you did from these two equations
\(H^2 = R^2 - 4\)

\(R+H = 2\sqrt{3}\)

This equation:
\(R^2 - 4 = 2\sqrt{3}\)

and then this
\(R = \frac{4}{\sqrt{3}}\)

Sure, look below

\(H^2 = R^2 - 4\) ...... (1)

\(R+H = 2\sqrt{3}\) ........ (2)

From (2), \(H = 2\sqrt{3} - R\)

Square the above equation to get, \(H^2 = R^2+ (2\sqrt{3})^2 - 2*R*2\sqrt{3}\)

----> \(H^2 = R^2+12- 4R\sqrt{3}\) ...(3)

and now from (1) and (3)

\(R^2 - 4 = R^2+12- 4R\sqrt{3}\) ----> \(4R\sqrt{3} = 16\) ----> \(R = \frac{4}{\sqrt{3}}\)

BTW, I am interested in an alternate way to solve this question.

Hope this helps.

Atendiendo a los valores de las abscisas de cada uno de los puntos (que son parte de una circunferencia), tenemos

11, 7 y 9, ordenando de menor a mayor se tiene: 7, 9 y 11.

Podemos imaginar que (7,a) y (9,a) y (11,a) son parte de una misma recta y dado que 9 esta exactamente equisdistante a 7 y 11, si trazamos una recta paralela al eje Y y que pase por (9,a), dicha recta necesariamente pasará por el centro de la circunferencia.

Así tenemos que la recta paralela al eje y y que pasa por (9,a) es perpendicular a la recta que pasa por los puntos (7,a), (9,a) y (11,a), ya que esta última recta es paralela al eje X.

Aplicando trazos que definen dos cuerdas que se intersectan en una circunferencia se tiene:

La cuerda formada por los puntos (7,0) y (11,0) es de longitud 4. Esta cuerda es intersectada por otra cuerda que pasa por (9,0) y (9, 2*sqrt (3)) de la cual no se conoce su longitud total. Dicha longitud estará dad por 2*sqrt(3) + x que corresponde al diametro de la circunferencia , y esta suma se forma exactamente en el punto de intersección de ambas cuerdas:

Luego tenemos 2 * 2 = (2*sqrt(3)) * X, así encontramos que X = 4/(2*sqrt(3))

A esta altura descarto la alternativa B y la alternativa E

Finalmente el diametro es (2*sqrt(3)) + 4/(2*sqrt(3)) = (4*3 + 4)/(2*sqrt(3)) = 16/(2*sqrt(3)) = 8/(sqrt(3))

ALTERNATIVA C

claudio hurtado
GMAT GRE SAT MATH Tutor in Chile
www.gmatchile.cl

Hi gmatchile

Please post your replies in English as well. GMAT is an exam in English and will thus help everyone (most of whom are not Spanish speakers!).

Thanks

Yep, sure thing. As usual, geometry tasks have a lot of ways of solving

We have three points \((9, \ 2\sqrt{3})\), \((7, \ 0)\), and \((11, \ 0)\)

We have line at bottom from 7 to 11 and equidistant point between them (9) on the height \(\ 2\sqrt{3}\)
So we can infer that this is isosceles or equilateral triangle

Let's check it by finding distance between points \((9, \ 2\sqrt{3})\) and \((11, \ 0)\)
\((11-9)^2 + (0-\ 2\sqrt{3})^2 = distance^2\) --> \(4 + 12 = 16\) --> distance = 4

Line at bottom has length 4 and this second line has the same length so we have equilateral triangle.

Radius of circle which circumscribe equilateral triangle = \(\frac{a}{\sqrt{3}}\) where a is side of the equilateral triangle

Side of triangle equal to 4 so radius equal to \(\frac{4}{\sqrt{3}}\) and diameter equal to \(\frac{4*2}{\sqrt{3}}\)

---------------------------

P.S. I don't know why, but I didn't met this formula in Manhattan or Veritas, maybe I overlook it but just in case I explain how we can find this formula by ourself:

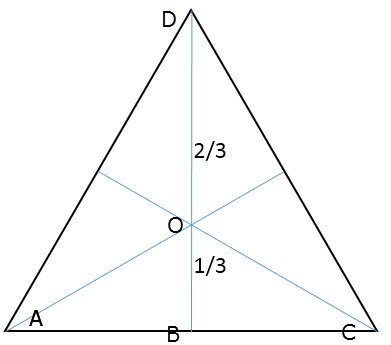
If we draw three heigths in equilateral triangle the intersection will be center of this triangle, also it will be center of circumsribed circle.
And as these heights split triangle symmetrically each height will be split on 2/3 and 1/3 parts



And this 2/3 is a radius of circumscribed circle. So if we know height we can multiple it on 2/3 ang received a radius of circumscribed circle.

I think this ratio 2/3 to 1/3 is quite intuitive so I decide to not write long proof with pythagorean triangles.

P.P.S radius of circle inscribed in equilateral triangle equal to \(\frac{a}{2*\sqrt{3}}\) where a is side of the equilateral triangle

It is not a new or separate formula but a derivative of a formula that you already know about. The ratio of sides for 30-60-90 triangle.

When you draw the perpendicular from the top point (9,2sqrt3) to the base , you create 2 congruent 30-60-90 triangles (Triangles DBA and DBC) with sides in the ratio: \(1:\sqrt{3}:2\)

Additionally, when you draw the radius of the circumcircle, the radius (OA) bisects \(\angle{DAB}\) such that \(\angle{OAB} = 30.\)

Thus, in triangle, AOB, \(\angle{OAB} = 30\), as the radius of the circumcircle will bisect the angles of an equilateral triangle (you can figure it out by symmetry as well!)

Thus, triangle AOB , becomes another 30-60-90 triangle with ratio \(1:\sqrt{3}:2\) .

So , if the the side "sqrt{3}" = 2 units,

then the radius = R = side with "2" = \(\frac{4}{\sqrt{3}}\).

The property of '2/3' or '1/3' is a property of equilateral triangles and its altitudes. The altitudes in an equilateral triangle meet at the orthocenter, which divides the altitudes (or height or perpendiculars to the sides!) in the ratio 2/3 and 1/3 with 2/3 closer to the vertex. This is not a usual property of triangles and is thus not mentioned in most of the GMAT quant books. There will always be another way to solve such questions.

As an additional fun fact about equilateral triangles, the circumcenter, the incenter (center of the incircle) and the orthcenter all lie at the same point.

Based on the values ​​of the abscissa of each of the points (that are part of a circumference), we have

11, 7 and 9, ordered from smallest to largest we have: 7, 9 and 11.

We can imagine that (7, a) and (9 a) and (11 a) are part of the same line and since 9 is exactly equisdistante 7 and 11, if we draw a parallel to the axis line Y and passing through (9 a), said straight necessarily pass through the center of the circle.

Thus we have the line parallel to the axis yy through (9 a) is perpendicular to the line through the points (7, a), (9 a) and (11 a), since the latter straight It is parallel to the axis X.

Applying two strings defining lines that intersect at a circle has:

The rope formed by points (7.0) and (11.0) is of length 4. This string is intersected by another rope through (9.0) and (9, 2 * sqrt (3)) of the which total length is not known. This length is dad for 2 * sqrt (3) + x which corresponds to the diameter of the circle, and this sum is formed exactly at the point of intersection of the two strings:

Then we have 2 * 2 = (2 * sqrt (3)) * X, so we find that X = 4 / (2 * sqrt (3))

At this stage rule out the option B and option E

Finally is the diameter (2 * sqrt (3)) + 4 / (2 * sqrt (3)) = (4 * 3 + 4) / (2 * sqrt (3)) = 16 / (2 * sqrt (3) ) = 8 / (sqrt (3))

ALTERNATIVE C

Claudio hurtado
GMAT GRE SAT Math Tutor in Chile
www.gmatchile.cl

This is a chalenging question. IMO the hardest part might be to figure out that this is a circle with inscribed eqilateral triangle ( once you conect the 3 points).
Once you do that it is easy to get to the radius and aftre to the diameter.

The formula for the radius will be \(sqrt3/3*a\) where a is 4 beacuse it is the length between 7 to 11.

so from there it turns that r is \(sqrt3/3*4\) and multiply by 2 to get diameter and that is answer chouce C

OR

once you draw the diagram and figure out it is clearly visiable that the dimatere should be little over 4, which is the side of the tirangle.
only option C does that.

but under test condition im not sure if this 2 solutions will popo out in my head

Drawing a diagram, we can see that the diameter can not be more than (2*2)*√3 and less than 2*2. So the answer would be C. 8/(√3).

In above question, if (7,0) and (11,0) are points on other end, then center point of circle connect two points and create isoseles triangle with ratio 1:1:√2

The radius is 4/√2. However, the answer is not 8/√2

I solved this using a simple sketch method. Here was my approach.

Step 1: From the given information, sketch an image of what is given (my sketch looked something like the below attached image).

Step 2: Evaluate the answer choices:
(A) Eliminate! We know that the diameter must be larger than any chord that does not pass through the circle center. Eliminate A because 4/sqrt(3) is less than 4.
(B) Eliminate! For the same reason that we eliminated (A) we can eliminate (B).
(C) Possible Answer Choice!
(D) Eliminate! Referencing our sketch know that 3.4 (2*sqrt(3)) is greater than the radius of the circle, so we know for sure that the diameter must be less than twice 3.4 (or diameter must be less than 6.8).
(E) Eliminate! For the same reason that we eliminated (D) we can eliminate (E).

Step 3: We know that C is the only viable answer choice!

I can't believe I actually found the correct answer for the Center, and then solved for the Radius at the end.

I forgot to double the Radius to get the Diameter.

Man, you're evil lol

The diameter must be longer than the red segment (4) but shorter than twice the blue segment (\(4\sqrt{3}\)).
Only C is viable.

Inference 1: the Point (9 , 2*sqrt(3) ) is Equidistant from Points (7,0) and (11,0) ---- these are All Points on the Circumference of the Circle.

Joining these 3 Given Points, we can Create a Triangle with the X-Axis as 1 of the Sides of the Triangle that is also a Chord of the Circle. The Triangle will be INSCRIBED Inside the Circle

Since the Vertex (9 , 2*sqrt(3) ) -----> is Equidistant from the Other Triangle Vertices (7,0) and (11,0) we know that at the very least these 2 Sides are Equal ---->

thus we can Infer that the Inscribed Triangle is an Isosceles Triangle (possibly Equilateral)


Inference 2: on the X-Axis, the Point (9,0) is right in the middle of the Other 2 Points on the Circumference of the Circle----> 2 units Away from (7,0) and (11,0)

RULE: the Height drawn from the Vertex between the 2 Equal sides of an Isosceles Triangle to the NON-Equal Side will always BISECT the NON-Equal Side

thus, we can drop the Altitude from the Vertex of the Triangle "Touching" the Circle at (9, 2*sqrt(3)) -----> to the Base of the Side created on the X-Axis from (7,0) to (11,0)

this creates TWO 90 degree Triangles


Inference 3: the TWO 90 Degree Triangles Each with the 90 Degree Vertex at Point (9 , 0) will EACH have its Legs-Sides in the Ratio of:

2 : 2*sqrt(3) --------> 1 : sqrt(3) --------> Given a Right Triangle, when we know the Legs of the Right Triangle are in this Ratio, the Right Triangle is a 30/60/90 Triangle

Thus, both Right Triangles we created are 30/60/90 Triangles

when you put TWO 30/60/90 Triangles together, you get ONE Equilateral Triangle.


Rule 1: the Largest Area-Triangle that can be Inscribed inside a Circle is an Equilateral Triangle.

Our Equilateral Triangle Inscribed in the Circle has its vertices at: (7,0) --- (9 , 2*sqrt(3) ) ------ (11 , 0)

the Side Length can be measured along the X-Axis from (7 ,0) -----> to (11 , 0)

thus showing us that the Side Length of the Inscribed Equilateral Triangle = 4



Rule 2: the Circum-Radius of an Inscribed Equilateral Triangle = (2/3) * (Altitude of the Equilateral Triangle)

This is because all 3 Altitudes of an Equilateral Triangle are Congruent, Axis of Symmetry that meet at the Same Geometric Center = Centroid = Orthocenter = Circum-Center


The Radius of the Circumscribed Circle around the Equilateral Triangle (called the Circum-Radius) = the Line from this Geometric Center - to - the Point of Tangency where the Equilateral Triangle's Vertex "Touches" the Circle that Circumscribes it


The Altitude-Height of our Inscribed Equilateral Triangle is given by the Distance along the Y-Axis from Point (9,0) -----> to Point (9 , 2*sqrt(3) )

Height = 2 * sqrt(3)


Circum-Radius = Radius of Circle = (2/3) * (2 * sqrt(3) ) = 4 * sqrt(3) * (1/3)

Double this Radius to get the Diameter of the Circle = 2 * [ 4 * sqrt(3) * (1/3) ] =



8 * sqrt(3) * (1/3) -----> which is the Answer



"UN-Conjugate" the Answer to get -----> 8 / (sqrt(3) )

or Conjugate -C- by Multiplying the NUM and DEN by * sqrt(3) to get the Answer Above

-C-



Awesome question!

Hi ENGRTOMBA2018, I am not sure but are you missing out something in the formula?
shouldn't it be H^2 = R^2 + (2\sqrt{3})^2 - 2*R*2\sqrt{3} -4 ?

Hello from the GMAT Club BumpBot!

Thanks to another GMAT Club member, I have just discovered this valuable topic, yet it had no discussion for over a year. I am now bumping it up - doing my job. I think you may find it valuable (esp those replies with Kudos).

Want to see all other topics I dig out? Follow me (click follow button on profile). You will receive a summary of all topics I bump in your profile area as well as via email.