Bunuel wrote:
The sum of all solutions for x in the equation x^2 – 8x + 21 = |x – 4|+ 5 is equal to:
(A) –7
(B) 7
(C) 10
(D) 12
(E) 14
Kudos for a correct solution.
MANHATTAN GMAT OFFICIAL SOLUTION:a. Unknowns: x
Given: x2 – 8x + 21 = |x – 4| + 5.
Constraints: The question implies that there may be multiple solutions, as does the non-linear given equation.
Question: What is the sum of all possible solutions for x?
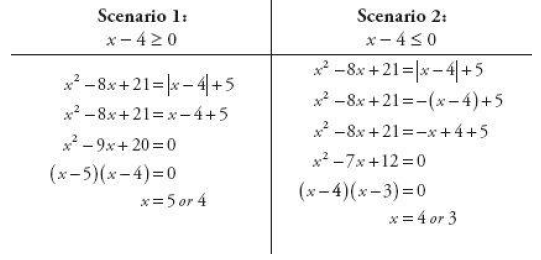
b. Break into 2 equations (i.e. non-negative and negative inside absolute value sign) → Group all variables on one side of the equation, with 0 on the other → Factorable quadratic? → Factor → Solve → Answer.
Attachment:
 2015-05-11_1802.png [ 55.23 KiB | Viewed 44606 times ]
2015-05-11_1802.png [ 55.23 KiB | Viewed 44606 times ]
Sum of the different solutions: 5 + 4 + 3 = 12.
The answer is D.Alternatively, we could focus on the left side of the equation, which looks like a manageable quadratic, isolating the absolute value on the right:
x^2 – 8x + 21 = |x – 4| + 5
x^2 – 8x + 16 = |x – 4|
(x – 4)(x – 4) = |x – 4|
(x – 4)2 = |x – 4|
“Something squared equals its absolute value. 1 squared equals 1, 0 squared equals 0…hmm, –1 squared equals 1…”
x – 4 = –1, 0, or 1
x = 3, 4, or 5
Sum of the different solutions: 5 + 4 + 3 = 12.
d. On absolute value problems, some of the solutions might be duplicates. Just cross these off at the end. Additionally, we could check to make sure the results for each solutions satisfy the initial assumptions. For example, in Scenario 1 we assumed x – 4 ≥ 0. Both solutions, 5 and 4, satisfy this inequality. Similarly, the solutions 4 and 3 satisfy Scenario 2’s assumption, that x – 4 ≤ 0. Thus all the solutions were valid. For complex absolute value equations such as this one, this need
not always be the case.



 45%
(medium)
45%
(medium)
 27%
(02:49)
wrong
27%
(02:49)
wrong  based on 2433
sessions
based on 2433
sessions







