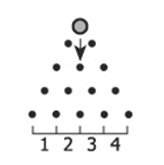
The figure shown represents a board with 4 rows of pegs, and at the bo

When ball passes two pegs it will be either on the left route or on the right route. Now, if ball is on the left route, number of possible scenarios will be 4, out of which two will lead to cell 2, if ball is on the right route, number of scenarios will also be 4, but out of these four only one will lead to cell 2. So, total 8 scenarios out of which 2+1=3 lead to cell 2, hence probability 3/8.

Answer: D.



I think it's from GMAT Prep, not OG.
_________________

not sure i woul have got this without making a mistake, but......

1st gate, left or right

if it goes left it can then go : LR or RL

if it goes right it can then go : LL

so

LLR
LRL
RLL

(1/2 * 1/2 * 1/2) * 3

= 3/8

think its 3/8.

8 ways the ball lands in any of the cells.
only 3 ways does the ball land in cell two

Does anyone know which OG question this is ?
I haven't come across this in either 10th or 12th edition or Quant Review 2nd edition !

If a question is accompanied by a helpful picture, you can sometimes arrive at the answer simply by visualizing what's said to be happening in the picture. Concentrate and picture the ball dropping through those openings, landing on the peg and then taking a right or a left direction. Note the direction as it is dropping- LLR, for example. You will notice that the ball can turn left and left and right OR left and right and left OR right and left and left in order to land on Cell 2. For "AND" you know you have to multiply and for "OR" you know you have to add. So (1/2*1/2*1/2) + (1/2*1/2*1/2)+ (1/2*1/2*1/2). That's 3/8!

Probability of going left of peg = 1/2
probability of going right of peg = 1/2

After passing the first row, ball will hit a peg. so the probability of going left or right is 1/2.
Assume that ball goes to left:

Then the favorable outcome can be ball first going left then going right or ball first going right and then going left. Hence probability of ball hitting cell 2 after taking first left is = 1/2*1/2 = 1/4.

Now assume that ball goes to right:

The favorable outcome after taking right will be to take 2 left on next two pegs.
hence probability of hitting cell 2 after taking first right is 1/4 *1/2 =1/8

So the total probability = 1/8+1/4 =3/8

Just a couple of generalization. As you have already known making generalization is important in order not to solve every GMAT question from the very beginning, in other words, to solve GMAT questions quicker.

The quickest way to calculate all possible outcomes is keeping in mind that there are 3 rows of pegs to pass, each row gives 2 ways. Thus, all possible outcomes = 2*2*2 = 2^3=8
How to calculate desirable (or preferred) outcomes the above posts have already showed.

Looking deeper into the question we can find out the Galton board (or Bean machine) that demonstrates normal distribution. Watching the below video sticks you to this principle!
[youtube]https://www.youtube.com/watch?v=6YDHBFVIvIs[/youtube]

Hi

Let's say ball be at spot 1 - 2nd peg .. Now from there it can reach Cell 2 through LRR ,RLR,RRL .
Now if ball be at spot 2 - 2nd Peg .. Now from there it can reach Cell 2through LRL,RLL.
Similarly if ball be at spot 3- 2nd Peg .. It can reach cell 2 through LLL.

SO summing up results in 6/8 . Please let me know what i am doing wrong here.

Responding to a pm:

Note that the ball does not jump from peg to peg, it falls between two pegs. So when you have 4 different arrows, they show only 2 different paths.

You can use symmetry in this question to solve it. By symmetry, we see that probability of coming to cell 1 = probability of coming to cell 4

Similarly, probability of coming to cell 2 = probability of coming to cell 3

probability of coming to cell 1 + probability of coming to cell 2 + probability of coming to cell 3 + probability of coming to cell 4 = 1
2*(probability of coming to cell 1) + 2*(probability of coming to cell 2) = 1


Finding the probability of coming to cell 1:
After the first slot (not the peg but the slot between pegs 1 and 2 in first row), it moves left (between pegs 1 and 2 in second row) or right (between pegs 2 and 3 in second row). It must move left. Probability = 1/2
After that, it must move left again. Probability = 1/2
Finally it must love left again to come at cell 1. Probability = 1/2

Total probability = (1/2)*(1/2)*(1/2) = 1/8

Putting this in equation above:

2*(1/8) + 2*probability of coming to cell 2 = 1

Probability of coming to cell 2 = 3/8
_________________

It seems to me that this a clasical pascal triangle, and you can notice to come to the position in the forth row there are 8 ways, 1+3+3+1=8 and teh posotiion that is asked in teh problem is by teh number 3, which means there are 3 ways to get there, so it is 3/8 as final answer. teh pis I attched you can figure out how teh triangle is made so it will be more clear how to come to the solutiomn to the problem.

Hope it helps

Hi Karishma,

I understand your calculation and the logic behind it except for the concept of symmetry mentioned above. I've read your post in symmetry https://www.veritasprep.com/blog/2011/10/quarter-wit-quarter-wisdom-linear-arrangement-constraints-part-ii/, but the post discusses mainly about left and right positions in linear arrangement. So, I didn't get it here why cell 1 and cell 4 are symmetrical. Could you please throw some light on it?

Thank you very much!

The symmetry here being talked about is both visual and prob wise..
if you look at the number of pegs on 1 and compare it to 4, it is symmetrical..
similarily if you look at the distribution of pegs over 2 and 3, they are same..

SAy you put a mirror right in center and take a view if what is in left side..
the image in mirror will be exactly what is on the right side..
_________________

Hi
another way to look at it is..

there are 4 rows..
the ball has 2 ways of moving-- to left or to right..
again when it falls in the peg in lower row, it can move to either direction- left or right ..
the final row again can have two ways
so TOTAL ways = 2*2*2 = 8 ways..

now ways the ball will fall in 1 = ways it will fall in 4..
and ways the ball will fall in 2 = ways it will fall in 3..

If you look at ways it can go in 1-
at every PEG, the ball has to move ONLY to left, so ways = 1*1*1 = 1 way

similarly in 4, the ball has to move ONLY to RIGHT so 1 way..

total ways for 3 and 2 = 8-2=6..
ways for each = 6/2 = 3..
prob of ball falling in 2 = 3/8

D
_________________

Hi,
So, it is true even when we have odd numbers of objects, right? In the example above, there are 4 cells, hence cell 1 and cell 4 is symmetrical, and cell 2 and cell 3 are symmetrical. If we have 5 cells, the third cell (i.e. cell 3) will not be symmetrical to any other cell, right? But cell 1 and cell 5 are symmetrical, and cell 2 and cell 4 are symmetrical. Correct?

Thank you!

Yes it will be correct, as there will be same set up of pegs on top of symmetrical pegs..
in ODD, it will be as you have found, except the middle, all other will have a pair..
_________________

Say you flip the image along a vertical axis - what do you get? The same image but now 1 is in 4's spot and 4 is in 1's spot.
2 is in 3's spot and 3 is in 2's spot.

The figure is still exactly the same though. The pathways in which you could reach slot 1 are the pathways in which you can reach slot 4 now.

OR think about it like this:
To reach slot 1, the ball needs to turn left-left-left. Probability = 1/2 * 1/2 * 1/2
To reach slot 4, the ball needs to turn right-right-right. Probability = 1/2 * 1/2 * 1/2
Since the probability of turning left or right is the same, the situations are symmetrical.

Same will be the case for slots 2 and 3.
_________________

At first here's a takeaway for you:
if you see a probability problem in which it's clear that there are relatively few possibilities, then, unless you IMMEDIATELY see a theoretical approach, you should open the problem by LISTING POSSIBILITIES.

whenever you make such a list, the list must of course be organized; if you make a haphazard list of possibilities as they randomly come into your head, with no organizing principle, then you will probably not assemble a complete list (and/or you will accidentally count possibilities more than one time each).

in this case, the ball goes either left or right whenever it hits a peg. this will happen three times between the release and the end of the trajectory, so you have a combination of three L's/R's.
here's an organized list, arranged by increasing numbers of L's (starting with no L's at all, working up to three L's):
LLL
LLR LRL RLL
LRR RLR RRL
RRR

here's another list, arranged alphabetically:
LLL
LLR
LRL
LRR
RLL
RLR
RRL
RRR

either way, you come to the conclusion that there are 8 possibilities, meaning that your probability fraction starts out with a denominator of 8. this is enough to eliminate choice (a), whose denominator is larger than 8, but that's about it.

now, the numerator.
this is the number of possibilities that actually get you into the desired slot.
an examination of the picture reveals that, to get into the desired slot #2, you have to go left exactly 2 times, and right exactly once, in some order. there are three possibilities in the above list that do this: LLR, LRL, and RLL.
therefore, probability = 3 out of 8.
--
THEORY APPROACH:
if you didn't think of this in about 15 seconds, then don't continue to look for it; it's here for your edification only. YOU SHOULD NOT KEEP LOOKING FOR THESE SORTS OF THINGS IF YOU DON'T FIND THEM RIGHT AWAY. REMEMBER THAT YOU SHOULD START LISTING RIGHT AWAY IF YOU DO NOT _IMMEDIATELY_ SEE THE THEORY APPROACH, AS THERE ARE NO POINTS FOR ELEGANCE OR STYLE.

the total number of possibilities is the total number of ways to have three L's or R's.
that's three decisions, with two options (L and R) at each decision.
therefore, the total number of possibilities is 2 x 2 x 2, or 8.

the total number of successes is the total number of ways to have two L's out of three.
this is a combination problem; the number of ways of picking two out of three is 3! / (2!1!), or 3.
alternatively, it's the total number of ways to pick one R out of three, which is clearly 3.

either way, 3 out of 8.

Answer is (D).
_________________

We can observe two points.

1. There are 4 rows of pegs. So the ball will have to go through 4-1 = 3 rows of pegs to reach the second cell on the bottom.

2. The second cell is situated on the left side. Since this cell is asymmetrically located on the left side, the ball will have more ways to reach the second cell from the left half of the figure than it has from the right half.

For the ball's travel through each level between two pegs, the probability is 1/2 (given) that the ball will take either the left or the right side. Since the ball has to travel through three rows of pegs, the combined probability of the ball reaching the second cell will be a multiple of (1/2)*(1/2)*(1/2). We are multiplying 1/2 three times because the ball will have to travel through three rows. For each travel through a row, we obtain a 1/2 probability.

Consider the left side. Here the ball has two ways to reach the second cell. So the probability here will be 2*(1/2)*(1/2)*(1/2)

Consider the right side. Here the ball has only one way to reach the second cell. This is because the second cell is asymmetrically located on the left side. So the probability here will be 1*(1/2)*(1/2)*(1/2).

The total probability of the ball reaching the second cell will be the simple sum of the above two probabilities.

This probability is equal to (2+1)*(1/2)*(1/2)*(1/2) = 3/8

ANSWER: D

I used POE here.

Since the board is symmetric, p2=p3 (p2 is the probability that the ball lands in cell 2 and so on)
p1=p4
=> 2p1+2p2=1
=>p1+p2=1/2
Given the structure, p2>1/4 and p2<1/2 (since p1=! 0)
only correct option is 3/8, hence D