As discussed by most of the experts here, this is a question which tests your knowledge of similarity concepts.
When you have a situation where a smaller triangle is inside a larger triangle, such that one side of the smaller is parallel to the corresponding side of the larger, corresponding angles will be equal and you will be able to prove the triangles similar.
Because the triangles are similar, their sides will be in the same ratio and so will be all the other line segments of the two triangles, like the altitudes, medians and angle bisectors.
Drawing a diagram for the first situation will give us a figure similar to this:
Attachment:
 29th May 2019 - Reply 4 - 1.JPG [ 14.1 KiB | Viewed 12202 times ]
29th May 2019 - Reply 4 - 1.JPG [ 14.1 KiB | Viewed 12202 times ]
Clearly, DE is parallel to BC, the sides AB and AC are the transversals. Angle A is common, angles ADE and ABC are corresponding angles and hence equal and so are angles AED and ACB. So, triangle ADE is similar to triangle ABC. Therefore,
\(\frac{DE}{BC}\) = \(\frac{AD}{AB}\)
\(\frac{x}{3}\) = \(\frac{(4-x)}{4}\)
which on solving gives us x = \(\frac{12}{7}\).
At this point, we do not have sufficient information to eliminate any of the options, so let us continue analyzing the second half of the question.
A diagram representing the second situation looks something like this:
Attachment:
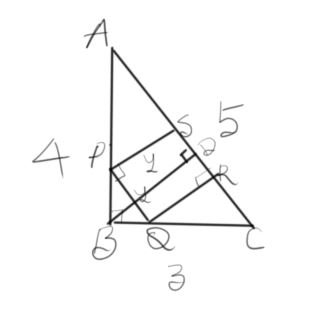 29th May 2019 - Reply 4 - 2.JPG [ 14.96 KiB | Viewed 11957 times ]
29th May 2019 - Reply 4 - 2.JPG [ 14.96 KiB | Viewed 11957 times ]
Using similar methods, we can prove that triangle BPQ is similar to triangle BAC (or ABC).
Area of triangle ABC = ½ * 3 *4 = ½ * 5 * BD. Therefore, BD = \(\frac{12}{5}\).
Now, since triangle BPQ is similar to triangle BAC,
\(\frac{Altitude of triangle BPQ}{BD}\) = \(\frac{y}{5}\) (because ratio of altitudes = ratio of corresponding sides).
Therefore, Altitude of triangle BPQ = BD * \(\frac{y}{5}\) = \(\frac{12}{5}\) * \(\frac{y}{5}\) = \(\frac{12y}{25}\).
Altitude of triangle BPQ + y = BD.
\(\frac{12y}{25}\) + y = \(\frac{12}{5}\).
Simplifying, we get, y = \(\frac{60}{37}\).
Therefore, \(\frac{x}{y}\) = \(\frac{12}{7}\) * \(\frac{37}{60}\) which simplifies to \(\frac{37}{35}\).
So, the correct answer option is D.
Hope that helps!

 95%
(hard)
95%
(hard)
 65%
(02:44)
wrong
65%
(02:44)
wrong  based on 68
sessions
based on 68
sessions






