PandaDude wrote:
Bunuel wrote:
PandaDude wrote:
When you square sqrt(b+2), doesnt it become |b+2|, and you can get two answers?
You would get 9^x +9^(-x) = b+2 OR = -b-4
No. I think you are mixing squaring and taking the square root.
\(\sqrt{x^2}=|x|\) but \((\sqrt{y})^2=y\).
Does this make sense?
Thanks for the response; I did not know that! I had assumed that since \(\sqrt{x^2}=(\sqrt{x})^2\)), that it would be the same in this case.
I just checked back on some notes, and this is not covered in any material I can find. Is there a "name/term" for this and where I can read up on this weird interaction?
i.e. \(\sqrt{x^2}=|x|\) but \((\sqrt{y})^2=y\), but also \(\sqrt{x^2}=(\sqrt{x})^2\)?
Two points before we move to your actual question:
1. All number on the GMAT are real numbers by default. This means that even roots (e.g. the square root, the fourth root, ...) are NOT defined for negative numbers on the GMAT. For example, \(\sqrt{-10}\) is not defined.
2. When we have the square root sign for an even root, such as a square root, fourth root, etc. then the only accepted answer is the non-negative root. That is:
\(\sqrt{9} = 3\), NOT +3 or -3;
\(\sqrt[4]{16} = 2\), NOT +2 or -2.
\(\sqrt{...}\) is the square root sign, a function (called the principal square root function), which cannot give negative result. So, this sign (\(\sqrt{...}\)) always means non-negative square root.
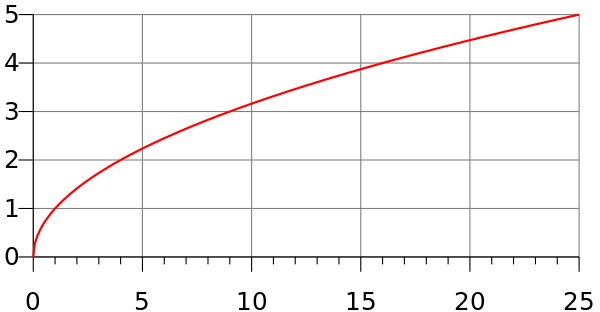 The graph of the function f(x) = √x
The graph of the function f(x) = √xNotice that it's defined for non-negative numbers and is producing non-negative results.
TO SUMMARIZE:
When the GMAT (and generally in math) provides the square root sign for an even root, such as a square root, fourth root, etc. then the only accepted answer is the non-negative root. That is:
\(\sqrt{9} = 3\), NOT +3 or -3;
\(\sqrt[4]{16} = 2\), NOT +2 or -2;
Notice that in contrast, the equation \(x^2 = 9\) has TWO solutions, +3 and -3. Because \(x^2 = 9\) means that \(x =-\sqrt{9}=-3\) or \(x=\sqrt{9}=3\).
Now, about \(\sqrt{x^2}=|x|\). Notice that the square root there gives, as it should, non-negative result: |x| (the absolute value of a number is always nonnegative). Consider this, say we have \(\sqrt{x^2}=5\). What is the value of x? Well, x can obviously be 5: \(\sqrt{5^2}=\sqrt{25}=5\) but it can also be -5: \(\sqrt{(-5)^2}=\sqrt{25}=5\). So, as you can see \(\sqrt{x^2}=5\) means that \(\sqrt{x^2}=|x|=5\), which gives x = 5 or x = -5.
Next, \(\sqrt{x^2}\) and \((\sqrt{x})^2\) are not exactly the same. For \((\sqrt{x})^2\) to be defined on the GMAT, x, which is under the square root, must be nonnegative, so if say, it's given that \((\sqrt{x})^2=5\), then x can only be 5. But if it's given that \(\sqrt{x^2}=5\), then x can be 5 or -5.
Hope it's clear.



 25%
(medium)
25%
(medium)
 21%
(02:05)
wrong
21%
(02:05)
wrong  based on 4539
sessions
based on 4539
sessions



