Events & Promotions
| Last visit was: 06 Sep 2026, 17:08 |
It is currently 06 Sep 2026, 17:08 |
|
|
GMAT Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
Sep 0712:01 AM PDT
-11:59 PM PDT
On the 7th, GMAT Club Tests will be Free! Including all the Quizzes, Questions, and Tests. 12 AM - 11:59 PM PDT- Sep 07
10:00 AM PDT
-11:00 AM PDT
A complete walkthrough of GMAT Club’s free 12-week GMAT study plan: what you study each week, how progress and goals are tracked, how the error log works, and how your practice unlocks paid tools at no cost. 
Sep 0201:00 PM EDT
-11:59 PM EDT
Take 30% off all study plans with code COURSEWORK - Expires on Monday 9/7
Sep 0410:00 AM EDT
-11:59 PM EDT
We’ve worked incredibly hard to build TTP into the best test prep experience possible, and it would mean a lot to us to win Newsweek’s 2026 Readers’ Choice Award for Best Test Prep. If TTP has helped you, we’d be incredibly grateful for your vote.
Sep 0609:30 AM EDT
-12:30 PM EST
Top scores are possible when you enroll in a powerful EA course, taught live online + 6 months access to TTP OnDemand video courses included! Perfect class schedule and easy course access for working professionals. Class starts Sundays Sept. 6, 2026.- Sep 09
07:00 AM PDT
-10:00 AM PDT
Meet AdComs and explore top Master’s programs - MiM, MiF, MSc, MSBA and more. - Application Fee Waivers - Free 1-Week of GMAT Club Tests: - Master's Application Toolkit - Grand Prize Giveaway - Sep 09
10:00 AM PDT
-11:00 AM PDT
Three MBA applications. Three rejections. No interview invites. A year later, Aman was admitted to Cambridge Judge, SMU, and IMD. In this episode of MBA Admit Stories, Aman shares how he rebuilt his MBA application... 
Sep 1507:00 PM EDT
-09:00 PM EST
Elite scores are possible when you enroll in a powerful GMAT course, taught live online + 6 months access to TTP OnDemand video courses included! Class starts Tues/Thurs Sept. 15, 2026 - Nov. 15, 2027, 7:00pm-9:00pm EST
Sep 2108:00 PM EDT
-10:00 PM EDT
Boost your GMAT score in less than one month in a live online class + 6 months access to TTP OnDemand video courses included! Class starts Mon, Tues, Wed, Thur, Fri Sept. 21, 2026 - Oct. 9, 2027, 7:00pm-10:00pm EST
03 May 2020, 10:51
Kudos
Bookmarks
C
Be sure to select an answer first to save it in the Error Log before revealing the correct answer (OA)!
Difficulty:
95%
(hard)
Question Stats:
42% (02:48) correct
58%
(03:05)
wrong
based on 77
sessions
History
Date
Time
Result
Not Attempted Yet
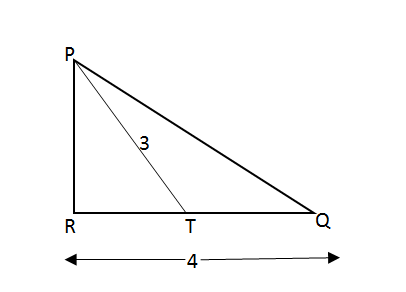
In a figure above, triangle PQR is right-angled at angle R. If the perimeter of triangle PQT equals to the perimeter of triangle PTR, then:
A. \(0 < \frac{QT}{TR} < \frac{1}{3}\)
B. \(\frac{QT}{TR} = \frac{1}{3}\)
C. \(\frac{1}{3} < \frac{QT}{TR} < 1\)
D. \(\frac{QT}{TR} = 1\)
E. \(\frac{QT}{TR} >1\)
Are You Up For the Challenge: 700 Level Questions
Attachment:
geo-41.png [ 7.41 KiB | Viewed 5892 times ]
firas92
Current Student
Joined: 16 Jan 2019
Last visit: 24 Jul 2026
Posts: 616
Own Kudos:
Given Kudos: 142
Location: India
Concentration: General Management
Schools: Tepper '23 (M$) Foster '23 (D)
GMAT 1: 740 Q50 V40
WE:Sales (Other)
Products:
Bunuel
Posted from my mobile device
03 May 2020, 13:05
Kudos
Bookmarks
Bunuel
Given: In a figure above, triangle PQR is right-angled at angle R.
Asked: If the perimeter of triangle PQT equals to the perimeter of triangle PTR, then:
Attachment:
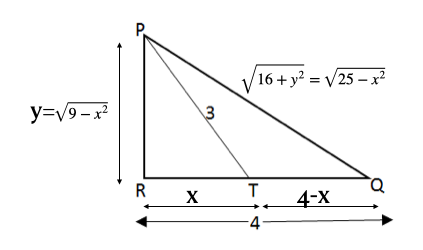Screenshot 2020-05-04 at 8.53.31 PM.png [ 24.95 KiB | Viewed 5276 times ]
Perimeter of triangle PTR = PR + RT + PT = PR + RT + 3
Perimeter of triangle PQT = PQ + TQ + PT = PQ + TQ + 3
Since the perimeter of triangle PQT equals to the perimeter of triangle PTR
PR + RT = PQ + TQ (1)
Let TR be x and and PR be y
PQ^2 = 4^2 + y^2 = 16 + y^2
Since x^2 + y^2 = 9
PQ^2 = 16 + 9 - x^2 = 25 - x^2
TQ = 4 - x
TQ + PQ = x + y = TQ + PQ = \((4-x) + \sqrt{25 - x^2}\)
\((2x + y - 4)^2 = 25 - x^2\)
\(4(x-2)^2 + 4(x-2)y + y^2 = 25 - x^2 \)
\(4x^2 - 16x + 16 + 4xy - 8y = 25 - 9 = 16\)
\(x^2 - 4x + xy - 2y = 0 \)
\(y = \frac{(x^2 - 4x)}{(2 -x)} >0\)
\(y = \frac{x(4-x)}{(x-2)} > 0 ; x>2\)
\(y^2 = 9 - x^2 = \frac{x^2 (4-x)^2}{(x-2)^2} = (3-x)(3+x) > 0 ; x < 3\)
2<x<3
\(\frac{QT}{TR} = \frac{(4-x)}{x} = \frac{4}{x} - 1\)
\(For\ x = 2 ; \frac{(4-x)}{x} = \frac{4}{2} - 1 = 1\)
\(For\ x = 3; \frac{(4-x)}{x} = \frac{4}{3} - 1 = \frac{1}{3}\)
\(\frac{1}{3} < \frac{QT}{TR} < 1\)
IMO C
06 May 2020, 22:03
Kudos
Bookmarks
Please See The Attached File
Since ∆ PQR is a right-angled triangle, \(a^{2}+ 16= b^{2}\) ---> \((b-a)(b+a )= 16\)
The perimeter of triangle PQT equals to the perimeter of triangle PTR:
---> \(a+x +3 = b+ (4-x) + 3\)
\(b -a = 2x -4 \)
---> \((b+a )= \frac{16}{(2x -4)}= \frac{8}{(x-2)}\)
One of features of ∆PRQ is \(b+a > 4\)
---> \( \frac{8}{(x-2)} >4\)
\(\frac{2}{(x-2)} > 1\)
\(\frac{(x-4)}{( x-2)} < 0\)
Since ∆ PTR is a right-angled triangle, \(x <3\).
So, we got x ----> \(x> 2\) and \(x <3 \)
Well,
\(x(2)= \frac{4-x }{x} = \frac{4-2}{2}= 1\)
\(x(3) =\frac{4-x }{x} = \frac{4-3}{3}= \frac{1}{3} \)
\(\frac{1}{3} < \frac{4-x}{x} < 1\)
Answer (C).
Since ∆ PQR is a right-angled triangle, \(a^{2}+ 16= b^{2}\) ---> \((b-a)(b+a )= 16\)
The perimeter of triangle PQT equals to the perimeter of triangle PTR:
---> \(a+x +3 = b+ (4-x) + 3\)
\(b -a = 2x -4 \)
---> \((b+a )= \frac{16}{(2x -4)}= \frac{8}{(x-2)}\)
One of features of ∆PRQ is \(b+a > 4\)
---> \( \frac{8}{(x-2)} >4\)
\(\frac{2}{(x-2)} > 1\)
\(\frac{(x-4)}{( x-2)} < 0\)
Since ∆ PTR is a right-angled triangle, \(x <3\).
So, we got x ----> \(x> 2\) and \(x <3 \)
Well,
\(x(2)= \frac{4-x }{x} = \frac{4-2}{2}= 1\)
\(x(3) =\frac{4-x }{x} = \frac{4-3}{3}= \frac{1}{3} \)
\(\frac{1}{3} < \frac{4-x}{x} < 1\)
Answer (C).
Attachments
right_angled.png [ 10.51 KiB | Viewed 5192 times ]
