3-D GeometriesThis post is a part of [
GMAT MATH BOOK]
created by: shrouded1ScopeThe GMAT often tests on the knowledge of the geometries of 3-D objects such cylinders, cones, cubes & spheres. The purpose of this document is to summarize some of the important ideas and formulae and act as a useful cheat sheet for such questions
CubeAttachment:
 cube.jpg [ 8.97 KiB | Viewed 54119 times ]
cube.jpg [ 8.97 KiB | Viewed 54119 times ]
A cube is the 3-D generalisation of a square, and is characterized by the length of the side, \(a\). Important results include :
- Volume = \(a^3\)
- Surface Area = \(6a^2\)
- Diagnol Length = \(\sqrt{3}a\)
CuboidAttachment:
 cuboid.jpg [ 16.29 KiB | Viewed 54195 times ]
cuboid.jpg [ 16.29 KiB | Viewed 54195 times ]
A cuboid is the 3-D generalisation of a rectangle, and is characterized by the length of its sides, \(a,b,c\). Important results include :
- Volume = \(abc\)
- Surface Area = \(2(ab+bc+ca)\)
- Diagnol Length = \(\sqrt{a^2+b^2+c^2}\)
CylinderAttachment:
 cylinder.jpg [ 24.95 KiB | Viewed 54036 times ]
cylinder.jpg [ 24.95 KiB | Viewed 54036 times ]
A cylinder is a 3-D object formed by rotating a rectangular sheet along one of its sides. It is characterized by the radius of the base, \(r\), and the height, \(h\). Important results include :
- Volume = \(\pi r^2 h\)
- Outer surface area w/o bases = \(2 \pi r h\)
- Outer surface area including bases = \(2 \pi r (r+h)\)
ConeAttachment:
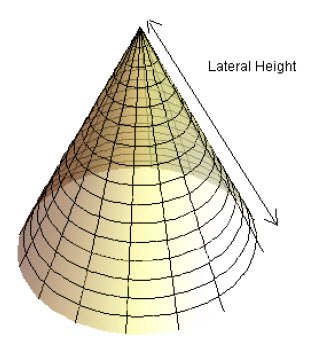 cone.jpg [ 31.94 KiB | Viewed 53919 times ]
cone.jpg [ 31.94 KiB | Viewed 53919 times ]
A cone is a 3-D object obtained by rotating a right angled triangle around one of its sides. It is charcterized by the radius of its base, \(r\), and the height, \(h\). The hypotenuse of the triangle formed by the height and the radius (running along the diagnol side of the cone), is known as it lateral height, \(l=\sqrt{r^2+h^2}\). Important results include :
- Volume = \(\frac{1}{3} \pi r^2 h\)
- Outer surface area w/o base = \(\pi r l =\pi r \sqrt{r^2+h^2}\)
- Outer surface area including base = \(\pi r (r+l)=\pi r (r+\sqrt{r^2+h^2})\)
SphereAttachment:
 sphere.jpg [ 32.94 KiB | Viewed 53756 times ]
sphere.jpg [ 32.94 KiB | Viewed 53756 times ]
A sphere is a 3-D generalisation of a circle. It is characterised by its radius, \(r\). Important results include :
- Volume = \(\frac{4}{3} \pi r^3\)
- Surface Area= \(4 \pi r^2\)
Attachment:
 hemisphere.jpg [ 36.64 KiB | Viewed 53657 times ]
hemisphere.jpg [ 36.64 KiB | Viewed 53657 times ]
A hemisphere is a sphere cut in half and is also characterised by its radius \(r\). Important results include :
- Volume = \(\frac{2}{3} \pi r^3\)
- Surface Area w/o base = \(2 \pi r^2\)
- Surface Area with base = \(3 \pi r^2\)
Some simple configurationsThese may appear in various forms on the GMAT, and are good practice to derive on one's own :
- Sphere inscribed in cube of side \(a\) : Radius of sphere is \(\frac{a}{2}\)
- Cube inscribed in sphere of radius \(r\) : Side of cube is \(\frac{2r}{\sqrt{3}}\)
- Cylinder inscribed in cube of side \(a\) : Radius of cylinder is \(\frac{a}{2}\); Height \(a\)
- Cone inscribed in cube of side \(a\) : Radius of cone is \(\frac{a}{2}\); Height \(a\)
- Cylinder of radius \(r\) in sphere of radius \(R\) (\(R>r\)) : Height of cylinder is \(2\sqrt{R^2-r^2}\)
ExamplesExample 1 : A certain right circular cylinder has a radius of 5 inches. There is oil filled in this cylinder to the height of 9 inches. If the oil is poured completely into a second right cylinder, then it will fill the second cylinder to a height of 4 inches. What is the radius of the second cylinder, in inches?
A. 6
B. 6.5
C. 7
D. 7.5
E. 8
Solution : The volume of the liquid is constant.
Initial volume = \(\pi * 5^2 * 9\)
New volume = \(\pi * r^2 * 4\)
\(\pi * 5^2 * 9 = \pi * r^2 * 4\)
\(r = (5*3)/2 = 7.5\)
Answer is (d)
Example 2 : A spherical balloon has a volume of 972 \(\pi\)cubic cm, what is the surface area of the balloon in sq cm?
A) 324
B) 729
C) 243 \(\pi\)
D) 324 \(\pi\)
E) 729 \(\pi\)
Solution : \(V=\frac{4}{3} \pi r^3\)
\(r = (\frac{3V}{4\pi})^{\frac{1}{3}} = (\frac{3 * 972 * \pi}{4 * \pi})^{\frac{1}{3}} = (3*243)^{1/3} = (3^6)^{1/3} = 9\)
\(A=4 \pi r^2 = 4 * \pi * 9^2=324 \pi\)
Answer (d)
Example 3 : A cube of side 5cm is painted on all its side. If it is sliced into 1 cubic centimer cubes, how many 1 cubic centimeter cubes will have exactly one of their sides painted?
A. 9
B. 61
C. 98
D. 54
E. 64
Solution : Notice that the new cubes will be each of side 1Cm. So on any face of the old cube there will be 5x5=25 of the smaller cubes. Of these, any smaller cube on the edge of the face will have 2 faces painted (one for every face shared with the bigger cube). The number of cubes that have exacly one face painted are all except the ones on the edges. Number on the edges are 16, so 9 per face.
There are 6 faces, hence 6*9=54 smaller cubes with just one face painted.
Answer is (d)
Example 4 : What is the surface area of the cuboid C ?
(1) The length of the diagnol of C is 5
(2) The sum of the sides of C is 10
Solution : Let the sides of cuboid C be \(x,y,z\)
We know that the surface area is given be \(2(xy+yz+zx)\)
(1) : Diagnol = \(\sqrt{x^2+y^2+z^2}=5\). Not sufficient to know the area
(2) : Sum of sides = \(x+y+z=10\). Not sufficient to know the area
(1+2) : Note the identity \((x+y+z)^2=x^2+y^2+z^2+2(xy+yz+zx)\)
Now we clearly have enough information.
\(2(xy+yz+zx) = 10^2 - 5^2 = 75\)
Sufficient
Answer is (c)
3-D Geometry Questions:
https://gmatclub.com/forum/geometry-3-d ... 75006.htmlSome Other 3-D ProblemsSphere & CubeSphere & CylinderCylinder & CuboidCylinder & Cuboid IICylinderCubeCube IIConeCube IIICylinderHemisphere





