ABSOLUTE VALUE(Modulus)
This post is a part of [
GMAT MATH BOOK]
created by: walkeredited by: bb,
Bunuel--------------------------------------------------------
This topic is included in
GMAT ToolKit App (iPhone/iPod Touch)
[
read more] [
AppStore]
--------------------------------------------------------
DefinitionThe absolute value (or modulus)
|x| of a real number x is x's numerical value without regard to its sign.
For example,
|3| = 3;
|-12| = 12;
|-1.3|=1.3Graph: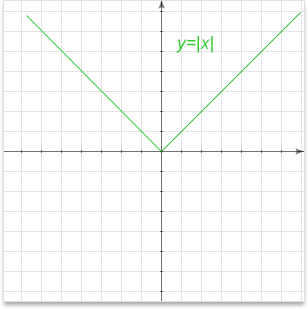
Important properties:|x|\geq0|x| = \sqrt{x^2}|0|=0|-x|=|x||x|+|y|\geq|x+y|3-steps approach:General approach to solving equalities and inequalities with absolute value:
1. Open modulus and set conditions.To solve/open a modulus, you need to consider 2 situations to find all roots:
- Positive (or rather non-negative)
- Negative
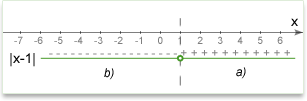
For example,
|x-1|=4a) Positive: if
(x-1)\geq0, we can rewrite the equation as:
x-1=4b) Negative: if
(x-1)<0, we can rewrite the equation as:
-(x-1)=4We can also think about conditions like graphics.
x=1 is a key point in which the expression under modulus equals zero. All points right are the first condition
(x>1) and all points left are second condition
(x<1).
2. Solve new equations:a)
x-1=4 --> x=5
b)
-x+1=4 --> x=-3
3. Check conditions for each solution:a)
x=5 has to satisfy initial condition
x-1>=0.
5-1=4>0. It satisfies. Otherwise, we would have to reject x=5.
b)
x=-3 has to satisfy initial condition
x-1<0.
-3-1=-4<0. It satisfies. Otherwise, we would have to reject x=-3.
3-steps approach for complex problemsLet’s consider following examples,
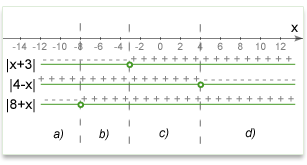
Example #1Q.: |x+3| - |4-x| = |8+x|. How many solutions does the equation have?
Solution: There are 3 key points here: -8, -3, 4. So we have 4 conditions:
a)
x < -8.
-(x+3) - (4-x) = -(8+x) -->
x = -1. We reject the solution because our condition is not satisfied (-1 is not less than -8)
b)
-8 \leq x < -3.
-(x+3) - (4-x) = (8+x) -->
x = -15. We reject the solution because our condition is not satisfied (-15 is not within (-8,-3) interval.)
c)
-3 \leq x < 4.
(x+3) - (4-x) = (8+x) -->
x = 9. We reject the solution because our condition is not satisfied (-15 is not within (-3,4) interval.)
d)
x \geq 4.
(x+3) + (4-x) = (8+x) -->
x = -1. We reject the solution because our condition is not satisfied (-1 is not more than 4)
(Optional) The following illustration may help you understand how to open modulus at different conditions.
Answer: 0
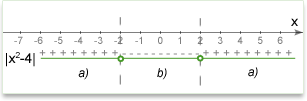
Example #2Q.: |x^2-4| = 1. What is x?
Solution: There are 2 conditions:
a)
(x^2-4)\geq0 -->
x \leq -2 or
x\geq2.
x^2-4=1 -->
x^2 = 5. x e {
-\sqrt{5},
\sqrt{5}} and both solutions satisfy the condition.
b)
(x^2-4)<0 -->
-2 < x < 2.
-(x^2-4) = 1 -->
x^2 = 3. x e {
-\sqrt{3},
\sqrt{3}} and both solutions satisfy the condition.
(Optional) The following illustration may help you understand how to open modulus at different conditions.
Answer:
-\sqrt{5},
-\sqrt{3},
\sqrt{3},
\sqrt{5}Tip & TricksThe 3-steps method works in almost all cases. At the same time, often there are shortcuts and tricks that allow you to solve absolute value problems in 10-20 sec.
I. Thinking of inequality with modulus as a segment at the number line.For example,
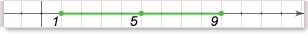
Problem: 1<x<9. What inequality represents this condition?
A. |x|<3
B. |x+5|<4
C. |x-1|<9
D. |-5+x|<4
E. |3+x|<5
Solution: 10sec. Traditional 3-steps method is too time-consume technique. First of all we find length (9-1)=8 and center (1+8/2=5) of the segment represented by 1<x<9. Now, let’s look at our options. Only B and D has 8/2=4 on the right side and D had left site 0 at x=5. Therefore, answer is D.
II. Converting inequalities with modulus into a range expression.In many cases, especially in DS problems, it helps avoid silly mistakes.
For example,
|x|<5 is equal to x e (-5,5).
|x+3|>3 is equal to x e (-inf,-6)&(0,+inf)
III. Thinking about absolute values as the distance between points at the number line.For example,
Problem: A<X<Y<B. Is |A-X| <|X-B|?
1) |Y-A|<|B-Y|
Solution: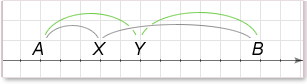
We can think of absolute values here as the distance between points. Statement 1 means than the distance between Y and A is less than that between Y and B. Because X is between A and Y, |X-A| < |Y-A| and at the same time the distance between X and B will be larger than that between Y and B (|B-Y|<|B-X|). Therefore, statement 1 is sufficient.
PitfallsThe most typical pitfall is ignoring the third step in opening modulus - always check whether your solution satisfies conditions.
Official GMAC Books:The Official Guide, 12th Edition: PS #22; PS #50; PS #130; DS #1; DS #153;
The Official Guide, Quantitative 2th Edition: PS #152; PS #156; DS #96; DS #120;
The Official Guide, 11th Edition: DT #9; PS #20; PS #130; DS #3; DS #105; DS #128;
Generated from [
GMAT ToolKit]
ResourcesAbsolute value DS problems: [
search]
Absolute value PS problems: [
search]
Fig's post with absolute value problems: [
Absolute Value Problems]
------------------------------------------------
Attachment:
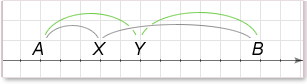lineAXYZ.png [ 8.44 KiB | Viewed 54738 times ]
Attachment:
line1x9.png [ 4.07 KiB | Viewed 45505 times ]
Attachment:
graph_modulus.png [ 7.61 KiB | Viewed 45636 times ]
Attachment:
Math_icon_absolute_value.png [ 1.78 KiB | Viewed 44172 times ]
Attachment:
Math_abs_example1.png [ 5.37 KiB | Viewed 44203 times ]
Attachment:
Math_abs_example2.png [ 3.67 KiB | Viewed 44119 times ]
Attachment:
Math_abs_example0.png [ 3.19 KiB | Viewed 44194 times ]
_________________
iOS/Android: GMAT ToolKit - The bestselling GMAT prep app | GMAT Club (free) | PrepGame | GRE ToolKit | LSAT ToolKit
PROMO: Are you an exiting GMAT ToolKit (iOS) user? Get GMAT ToolKit 2 (iOS) for only $0.99 (read more)
Math: GMAT Math Book ||| General: GMATTimer ||| Chicago Booth: Slide Presentation
The People Who Are Crazy Enough to Think They Can Change the World, Are the Ones Who Do.
close
