In the figure above, what is the value of x + y ?

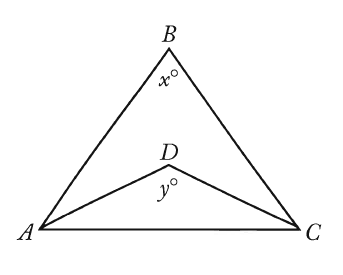In the figure above, what is the value of x + y ?

(1) x = 70.
(2) ABC and ADC are both isosceles triangles.

Even when we consider both statements together we don't know the placement of points D and B. For example consider the diagram below:

As you can see we can have two different answers for x+y for two different placements of point D.

Answer: E.


_________________

Bunuel and others,
I feel this question has a significant gap --- is the original diagram drawn to scale or not?

Of course, on the GMAT, we know "Figures are drawn as accurately as possible. Exceptions will be noted." I discuss this in a blog post.
https://magoosh.com/gmat/2012/gmat-trick ... -possible/
This is a crucial fact for students to keep in mind when interpreting GMAT diagrams.

IF this diagram is purporting to be drawn as accurate as possible, then it's an exceptionally poor diagram. That angle looks nothing like a 70 degree angle. If it's drawn to scale, though, we have to accept that the diagram is somewhat close to symmetrical, and therefore, the 70-70-40 triangle would not be possible.

IF the diagram is not drawn to scale, which I suspect was the intent of the author, that needs to be explicitly stated. Then, the 70-70-40 triangle would be possible. Any bilateral symmetry is out the window if it's not drawn to scale. Here's a scaled diagram of the figure with the 70-70-40 triangle.


I believe, either way, the answer would be (E). Nevertheless, I think this is a crucial issue for students to consider while analyzing the possibilities for a given diagram on the GMAT.

What do others think?
Mike
_________________

Very important point Mike. I also presumed ( from the diagram in the question that Ab=BC and AD=DC. So how do we tackle such questions in real exam ? Do we not take it scale.

Dear himanshujovi,
I'm happy to respond.

On GMAT PS, unless noted, problems are always drawn to scale. Of course, things that look equal may be close by not exactly equal, things that look parallel or perpendicular may be not exactly, etc. For such exact things, you need to rely on what the problem explicitly tells you.

Geometry on the GMAT DS is much trickier, because the diagram could be drawn to scale or not even pretending to be drawn to scale. You need to have a strong visual imagination. One way to approach this --- given a rough DS diagram and the constraints in the question, practice drawing on paper as many variants as possible that are still totally consistent with the explicitly given constraints. It takes some practice to develop your visual intuition, but the more your practice, the more you will be able to see different geometric possibilities.

If, for a specific problem, you would like to see some geometric variants, then post the problem as a new thread in the Magoosh forum:
magoosh-324/
and I will post some diagrams of possible altenatives.

Mike
_________________

OG13, page 272:
A figure accompanying a data sufficiency problem will conform to the information given in the question but will not necessarily conform to the additional information given in statements (1) and (2).
Lines shown as straight can be assumed to be straight and lines that appear jagged can also be assumed to be straight.
You may assume that the positions of points, angles, regions, and so forth exist in the order shown and that angle measures are greater than zero degrees.
All figures lie in a plane unless otherwise indicated.

OG13, page 150:
Figures: A figure accompanying a problem solving question is intended to provide information useful in solving the problem. Figures are drawn as accurately as possible. Exceptions will be clearly noted. Lines shown as straight are straight, and lines that appear jagged are also straight. The positions of points, angles, regions, etc., exist in the order shown, and angle measures are greater than zero. All figures lie in a plane unless otherwise indicated.

Hope it helps.
_________________

Hi Bunuel,

If they had provided us additional information as such: AB=BC, AD=AC and ADC = 1/2 height of ABC. If that's the case, can we use the same inscribed angle rules we use for circle - can we assume that Y would've been 2x, therefore 140 degrees?

Would the answer change if AB & BC weren't equal and AD & AC weren't equal?

Thanks

Dear russ9,
I'm happy to answer this.

If we knew AB=BC and AD=AC, then we would know the triangles were isosceles, which would have added a tremendous amount of useful information to the problem.

Your application of the inscribed angle rule, unfortunately, is 100% incorrect. Among other things, if triangle ABC were inscribed in a circle, the center of that circle would NOT lie on side AC. If ADC = 1/2 height of ABC, then that would NOT mean that AD bisected angle BAC or that CD bisected angle BCA. If we had been told that those two segments were angle bisectors, then yes, it would have been true that y = 140.

Here's a real paradox: if we had been told the three pieces of information you suggested, those would uniquely determine triangle ADC, such that all of its angles would be mathematically determined. To find the measure of y would involves some sophisticated trigonometry and a calculator --- finding the numerical value of y would be well beyond anything the GMAT could expect you to do, but technically, y would be mathematically determined and one could find it, which means that information would be sufficient. In practice, the GMAT doesn't put you in that position --- it doesn't expect you to find as sufficient something that is uniquely determined mathematically but incalculable using ordinary GMAT math. The GMAT will only put you in the position of declaring something sufficient if you realistically could find it with ordinary GMAT math.

Does all this make sense?
Mike
_________________

Hi Mike,

Thanks for the detailed response -- makes a lot of sense.

One point i'd like to bring up because I think that my original question wasn't phrased accurately:

1) If they give us that the height of ADC is 1/2 the height of ABC, doesn't that imply that the angle is doubled since AC is the same for both triangles? I meant to ask this and not bring in the "inscribed triangle" rule

Thanks!

Dear russ9
Absolutely and 100% NO!! I don't know how to be any more clear about this. You are laboring under a very powerful geometry misconception. There is no easy proportional relationship between distances and angles. If it were, trigonometry would be such a ridiculous easy topic that teachers could cover it entirely in a day toward the end of a geometry class. Instead, trigonometry is such a difficult topic that it requires almost a full year on its own. If you have never learned trigonometry, suffice to say there are all kinds of subtleties about the relationship of lengths and angles that you do not and cannot appreciate. That's OK. You don't need to know any of that for the GMAT. All you have to do is rid yourself of this pernicious misconception.

Consider this diagram:

Triangle ABC is a 70-55-55 isosceles triangle. Point D is the midpoint of altitude BE, and the angle at D is not a nice even 140 degrees. Instead, it's an irrational angle, that is, an angle whose measure is an irrational number. This is very typically the case in trigonometry: for example, triangles with all integer sides must have at least two angles that have irrational measures, and triangles with integer angle measures almost always have at least two sides that have irrational lengths. Again, all this is much more than you need to know. I simply want to impress upon you that there are absolutely no magic proportions that link the behavior of lengths and the behavior of angles.

Does all this make sense?
Mike
_________________

Hello avigutman ,

Please vet my below reasoning :-

S1 : X = 70

Hence angle BAC + angle BCA = 110 ( 180-70)


S2 :ABC and ADC are both isosceles triangles

Since ABC is an isosceles triangle , angle BAC = angle BCA
Also ADC is an isosceles triangle , angle DAC = angle DCA

Combining 1 + 2
In triangle ABC , angle BAC = angle BCA = 55 degree
Also,
Since angle DAC has to be equal to angle DCA , hence lines DA and DC are angle bisectors.
Consequently ,
angle DAC = 55 /2
angle DCA = 55/2
or
angle DAC + angel DCA = 55.

Now , in triangle DAC ,
55 + y = 180
Hence , y = 125

Thanks.

I boldfaced and highlighted your mistake above, PriyamRathor.
The way I quickly get to (E) is by visualizing the degree of freedom still available with both statements combined: point D can be dragged up or down without violating any of the constraints presented in the statements, yet any vertical movement of point D would change the answer.