GMAT Club World Cup 2022 (DAY 6): A poll conducted on the Wembley Stad

you don't need 1) or 2). Fans of Messi and those of CR7 are mutually exclusive. uh ah!

GMAT-wise, the answer should be E.

1) tells us that all Mbappe fans are Messi fans
2）tells us that out of all Messi fans, DeBruyne fans = 5 Mbappe fans (which is 1/6 of all Messi fans). So DeBrune fans + Mbappe fans = all Messi fans. Then we know 5/6 Messi fans are also DeBuyne fans.

We also know that 3/4 of DeBuyne fans are CR7 fans.

If we draw a circle for Messi fans and a circle for DeBuyne fans, the overlap is 5/6 of the Messi circle. But the remainder of DeBuyne circle is unknown. The CR7 circle can be anywhere- whether it overlaps with Messi circle is unknown.

Let n(Messi) be m, n(Mbappe)=o, n(DeBruyne)=p and n(Ronaldo)=q

S1:
"There is not a single Mbappe fan who is also not a fan of Messi."
Thus
n(Messi)=m
n(Mbappe)=m/6
n(DeBruyne)=(2/5)*(M/6)+k [k=fans of DB not fans of MB]
n(Ronaldo)=3/4[(2/5)(M/6)+k]+l [l=fans of Ronaldo not fans of DB]=q
Since no relationship between m and q
So INSUFFICIENT

S2:
"For every Messi fan, who is also a fan of Mbappe, there are 5 Messi fans, who are also fans of De Bruyne."
n(Messi)=m
n(Mbappe)=m/6+a [a=fans of Mbappe not fans of Messi]
n(DB)=(5m/6)+b[b=fans of DB not fan of Messi]
Since we have unknown variables and b, we cannot determine a relation between m and q
So INSUFFICIENT

S1+S2:
Please Refer to the attachment for Diagram
With all conditions applied we can arrive at two possibilities when n(Ronaldo) is disjoint from n(Messi) and also a case when n(Ronaldo) and n(Messi) are overlapping. So no clear relation between m and q. This INSUFFICIENT.

Imo E

1/6 of Messi fans = fans of Mbappe,
2/5 of Mbappe fans = fans of De Bruyne
3/4 of De Bruyne fans = fans of Ronaldo.
To find: The probability that a random Messi fan on the stadium, is also a fan of Ronaldo ?
Statement 1: There is not a single Mbappe fan who is also not a fan of Messi.
Using Vein diagram we can make a bigger circle for Messi and an incircle for Mbappe.
1/6 of Messi fans = Mbappe
2/5 of Mbappe = Fans of De Bruyne
3/4 of De Bruyne fans = fans of Ronaldo.
It is possible that 2/5 of Mbappe (or 1/6 Messi) doesn't overlap with the 3/4th De bruyne fans, which are also fans of Ronaldo. Hence the probability can be 0 or more.
Insufficient

Statement 2 For every Messi fan, who is also a fan of Mbappe, there are 5 Messi fans, who are also fans of De Bruyne.
1/6 of Messi fans (x): 5 De Bruyne fans.
Let's say there are 10 messi fans, who are also Mbappe fans
Then there will be 50 messi fans who are also De Bruyne's fans
But De Bruyne may have 200 fans and 3/4th of them doesn't have these 50 fans.
Insufficient

Combining both the statements
Again we don't know the relation between De Bruyne and Mbappe, who are also Ronaldo's fans
Insufficient

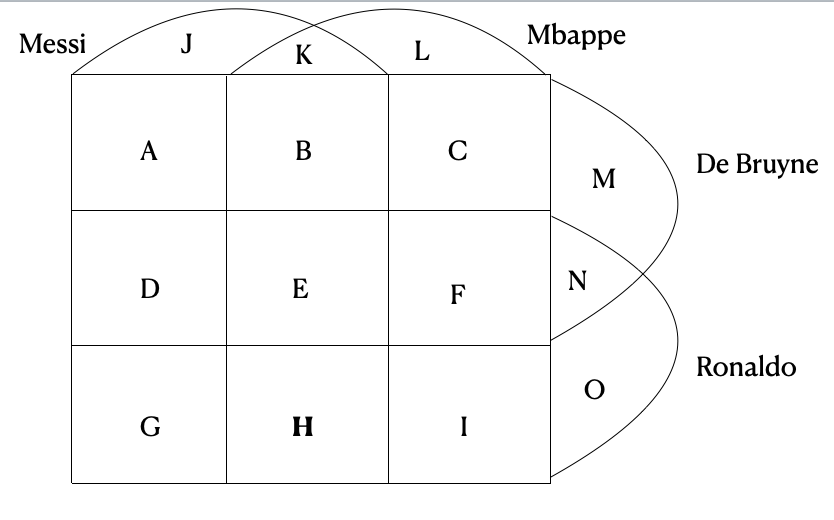
Given: A poll conducted on the Wembley Stadium revealed that 1/6 of Messi fans are also fans of Mbappe, 2/5 of Mbappe fans are also fans of De Bruyne, and 3/4 of De Bruyne fans are also fans of Ronaldo.

1. 1/6 of Messi fans are also fans of Mbappe,
(J + A + D + G + K + B + E + H) /6 = (K + B + E + H)
J + A + D + G = 5 (K + B + E + H)

2. 2/5 of Mbappe fans are also fans of De Bruyne
2/5 ( K + B + E + H + L + C + F + I) = B + C + E + F
2 (K + L + H + I ) = 3 (B + C + E + F)

3. 3/4 of De Bruyne fans are also fans of Ronaldo.
3/4 ( A + B + C + M + D + E + F + N ) = D + E + F + N
3 ( A +B + C+ M) = D + E + F + N


Asked: What is the probability that a random Messi fan on the stadium, is also a fan of Ronaldo ?

The probability that a random Messi fan on the stadium, is also a fan of Ronaldo = ( D +E + G+ H)/ (J + K + A + B + D + E + G + H)


(1) There is not a single Mbappe fan who is also not a fan of Messi.
L = C = F = I = 0
J + A + D + G = 5 (K + B + E + H)
2 (K + H ) = 3 (B + E )
3 ( A +B + M) = D + E + N
The probability that a random Messi fan on the stadium, is also a fan of Ronaldo = ( D +E + G+ H)/ (J + K + A + B + D + E + G + H)
Since there are 11 variables and 3 equations, it is NOT possible to find the probability.
NOT SUFFICIENT

(2) For every Messi fan, who is also a fan of Mbappe, there are 5 Messi fans, who are also fans of De Bruyne.
5 (K + B + E + H) = A + B + D + E
5 (K + H) = A + D
J + A + D + G = 5 (K + B + E + H) = A + D + 5(B + E)
J + G = 5 (B+E)
2 (K + L + H + I ) = 3 (B + C + E + F)
3 ( A +B + C+ M) = D + E + F + N
Since there are 15 variables, and 4 equations, it is NOT possible to find the probability.
NOT SUFFICIENT

(1) + (2)
(1) There is not a single Mbappe fan who is also not a fan of Messi.
L = C = F = I = 0
(2) For every Messi fan, who is also a fan of Mbappe, there are 5 Messi fans, who are also fans of De Bruyne.
5 (K + B + E + H) = A + B + D + E
5 (K + H) = A + D
J + A + D + G = 5 (K + B + E + H)
J + G = 5 (B + E)
2 (K + H ) = 3 (B + E )
3 ( A + B + M) = D + E + N
Since there are 11 unknowns and 4 equations, it is NOT possible to find the probability
NOT SUFFICIENT

IMO E

[quote="Bunuel"]A poll conducted on the Wembley Stadium revealed that 1/6 of Messi fans are also fans of Mbappe, 2/5 of Mbappe fans are also fans of De Bruyne, and 3/4 of De Bruyne fans are also fans of Ronaldo. What is the probability that a random Messi fan on the stadium, is also a fan of Ronaldo ?


(1) There is not a single Mbappe fan who is also not a fan of Messi.

(2) For every Messi fan, who is also a fan of Mbappe, there are 5 Messi fans, who are also fans of De Bruyne.

Suppose Number of Messi fans=a
Mbappe fans=b
Debruyne fans=c
Ronaldo fans=d
1) Now there is not a single MBAppe fan who is not a fan of Messi.
Thus Number of Mbappe fans=a/6=Total number of mbappe fans=b
Total number of Debruyne fans=2/5*a/6+p [p= number of debruyne fans who are not mbappe fans]
c=2/5*a/6+p
Total number of Ronaldo fans= 3/4[2/5*a/6+p]+q [q= number of Ronaldo fans who are not Debruyne fans]
As we cannot solve p and q ,thus 1 is insufficient.
2) For every Messi fan, who is also a fan of Mbappe, there are 5 Messi fans, who are also fans of De Bruyne.
Total number of Messi fans=a
Total Number of Mbappe fans=a/6 + x [ x= fans of mbappe who are not fans of mbappe]
Total number of Debruyne fans=5*a/6+y[y=fans of Debruyne who are not fans of Messi]
As we cannot determine x and y ,thus 2 is insufficient.

Now Combining 1 and 2
All Mbappe fans are Messi fans ,thus total number of Mbappe fans =a/6
Total Number of DeBruyne fans are 5/6*a+y=a/15+p
As we are not sure about the values of y and p.Thus combining both also this is insufficient.
Thus E is the answer.

Statement 1

Statement 1 is not sufficient as it does not provide us with any information on the overlap of the fans of Messi and De Bruyne.

Hence we can eliminate A and D

Statement 2

We do not know the overlap between Ronaldo and Messi. Hence we can eliminate this statement

Combining

We know that fans of Mbappe is a subset of the fans of Messi, and whoever is not a fan of Mbappe is a fan of De Bruyne.

Also, 2/5 of Mbappe fans are also fans of De Bruyne, and 3/4 of De Bruyne fans are also fans of Ronaldo.

However, this doesn't clarify how the overlap between De Bruyne and Ronaldo is, for instance the 1/4 can represent fans of De Bruyne who are fans of Messi or can also represent who are not a fan of messi. Hence we cannot find the overlap with the given information.

IMO E

Statements 1+2:
All Mbappe fans are fans of Messi.
So, Total Mbappe fans=\(\frac{1}{6}\)*Total Messi fans
Common fans of De Bruyne and Mbappe=\(\frac{2}{5}\)
Therefore, we can know the common fans of De Bruyne and Messi.
However, we don't know how many total fans does De Bruyne have and we are given the relationship of common fans of total De Bruyne and total Ronaldo.
Hence, we cannot find out, even after combining both statements, that how many fans are common between Messi and Ronaldo.
Hence, answer E.

total messi fans = a; total de bruyne fans = c
total mbappe fans = b; and total ronaldo fans =d

st. 1

from the question we know that a/6 are also fans of mbappe, from statement 1 we know that b = a/6

we also know that (b intersection c ) = 2b/5, which can be written as a/15

also, know that ( c intersection d ) = 3c/4

now since we don't know the relation between a, and d thus st. 1 alone is not sufficient. Also we don't know what is the relationship

st. 2

for every messi fan who is fan of mbappe (= a/6) there are 5 de bruyne fans, thus

thus (a intersecton b intersection c) = 5a/6,

since we don't know the relationship between a, and d thus st 2 alone is not sufficient

combining 1 and 2 we still don't know the relationship between ronaldo, and messi fans hence answer is E.

A poll conducted on the Wembley Stadium revealed that 1/6 of Messi fans are also fans of Mbappe, 2/5 of Mbappe fans are also fans of De Bruyne, and 3/4 of De Bruyne fans are also fans of Ronaldo. What is the probability that a random Messi fan on the stadium, is also a fan of Ronaldo ?


(1) There is not a single Mbappe fan who is also not a fan of Messi.

Not sufficient !! we can just say that 1/15 of Mbappe fans are also fans of De Bruyne

(2) For every Messi fan, who is also a fan of Mbappe, there are 5 Messi fans, who are also fans of De Bruyne.

Not sufficient !!

Statement 1 + statement 2

Also Not sufficient !!

IMO Option E

Let's say we have the below numbers
Messi = 180 fans
Mbappe = A + 30 fans (here A are non-Messi fans)
DeBruyne = B + (A + 30)*2/5 ( here B are non-Mbappe fans)
Ronaldo = C + ( B + (A + 30)*2/5 )*3/4 ( here C are non-DeBruyne fans)

(1) There is not a single Mbappe fan who is also not a fan of Messi.
This implies A = 0
=> Mbappe = 30 fans, who are also Messi fans
=> DeBruyne = B + (30)*2/5 = B + 12 fans
=> Ronaldo = C + (B + 12) *3/4
Since we don't know either B or C we cannot be sure of the exact probability of total fans that are Messi fans

(2) For every Messi fan, who is also a fan of Mbappe, there are 5 Messi fans, who are also fans of De Bruyne.
Since we have considered a total of 180 fans for Messi out of which 30 are Mbappe fans we have 150 De Bruyne fans
=> Mbappe = 30 + Z (here Z could be non-Messi fans)
=> DeBruyne = 150 + (30 + Z)*2/5 = 162 + Z*2/5
=> Ronaldo = C + 162 + Z*2/5
Since we don't know either Z or C we cannot be sure of the exact probability of total fans that are Messi fans

Using statements 1 and 2 together we get
Messi = 180 fans
=> Mbappe = 30
=> DeBruyne = 150 + 12 + M ( here M = Only DeBruyne fans or Only Ronaldo and DeBruyne fans )
Now 3/4 of DeBruyne fans are also Ronaldo fans => 3/4(150 + 12 + M)
However, there is no way to find out how many of the 162 Messi fans are included in the 3/4th of DeBruyne fans who are Ronaldo fans

IMHO Option E