![]() Rational Irrationality on the GMAT
Rational Irrationality on the GMAT
By: Eli Meyer
Despite the name, irrational numbers aren’t crazy. You might think they are, and you wouldn’t be alone. The followers of Pythagoras (yes, the right triangle guy!) believed that irrational numbers were heretical; supposedly, they threw the first person to prove irrationals existed into the ocean! But in fact, the word ‘irrational’ refers to the mathematical concept of the ‘ratio.’ Irrational numbers are numbers that cannot be expressed at the ratio of two integers, a/b.
There are an infinite number of irrational numbers, though far fewer are of mathematical note. If you’ve ever studied statistics or calculus, you’ve probably run across the natural number e, and ancient architects had a centuries-long love affair with φ, the golden ratio. But on the GMAT, there are only two types of irrational numbers that will be relevant to you: π and radicals.
Both of these irrationals will appear most often in geometry questions, and both of them have the same basic rule: you don’t need an exact numerical value. Because they can’t be reduced to a ratio (assuming, in the case of a radical, that the number underneath isn’t a perfect square), their values can’t be effectively determined with pen and paper. So generally, they will simply appear as-is in the answer choices. An answer choice to a circle problem will take the form of 3π, not 9.42.
As a result, we can treat these irrational terms just like we would a variable like an x or y. So on complex algebraic statements that feature irrational numbers, we can simplify by adding coefficients to irrationals and combining like terms. In other words, if we are given:
(3 X √2) + √2
we can express the first term with an algebraic coefficient:
3 √2 + √2
Then, we can combine like terms, getting:
4√2
Not only is this helpful and efficient, it’s necessary; GMAT answer choices will almost invariably be written in the simplest terms possible.
Similarly, Irrationals can (and sometimes must) be factored out as well. If the GMAT gives us a multi-irrational tangle like this one:
3π+π√2
Then anything we can do to make it more manageable will help us find the correct answer choice. In this case, we are adding two terms that are both multiplied by π. That means we can pull the π out. The result is a little simpler:
π (3+√2)
This could help us find the answer to a tough problem with circles and triangle. So get comfortable manipulating these indivisible terms, and you’re one step closer to acing the GMAT quantitative section.
Problem
A right circular cylinder has a height of 20 and a radius of 5. A rectangular solid with a height of 15, and a square base is placed in the cylinder such that each of the corners of the solid is tangent to the cylinder wall. Liquid is then poured into the cylinder such that it reaches the rim. What is the volume of the liquid?
(A) 500(π – 3)
(B) 500(π – 2.5)
(C) 500(π -2)
(D) 500(π – 1.5)
(E) 500(π- 1)
Solution
Step 1: Analyze the Question
For any Geometry question without a figure, our first step must always be to draw a quick sketch. This is particularly important for solids and complex word problems such as this question. The challenging element of this question is that a square solid is inscribed inside the right circular cylinder. Our sketch shows this:
Notice that we drew the radius of the circle to end at the corner where the circle and square meet. When working with multiple shapes, look for points or sides where the two shapes overlap, since you can use the common side or point to connect the equations of the shapes.
Step 2: State the Task
We must determine the volume of the liquid that has filled the cylinder. The volume of the liquid will be equal to the volume of the cylinder minus the volume of the rectangular solid. So we must calculate the volume of the cylinder and the volume of the rectangular solid.
Step 3: Approach Strategically
The volume of the cylinder is
To calculate the volume of the rectangular solid, we’ll need to determine the lengths of the sides of the square. We can draw a right triangle to help.
Recognizing a 45-45-90 triangle (always true of a bisected square), we know that the ratio of the side lengths of the triangle and can solve for the value of x because we know the hypotenuse is 10.
We can now calculate the volume of the liquid: Volume of Liquid = Volume of the Cylinder – Volume of the Rectangular Solid
Step 4: Confirm Your Answer
Reread the question, making sure that you did not misread any important information.

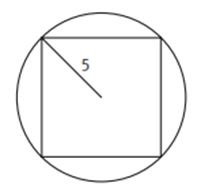



It is known that for a quadrilateral, in which the diagonals are perpendicular, the area is given by half the product of the diagonals. So, the area of a square is half the diagonal squared. This can shorten the calculations in the given example, as we already have the diagonal of the square (is the diameter of the circle).