How To Solve: Equation of a Line
Attached pdf of this Article as SPOILER at the top! Happy learning! 
Hi All,
I have recently uploaded a video on YouTube to discuss
Equation of a Line in Detail:
Following is covered in the video
¤ Equation of a Line: Two Point Form
¤ Equation of a Line: Point and Slope Form
¤ Equation of a Line: Intercept Form
¤ Generic Equation of a line (Point and Intercept Form)
¤ Equation of Horizontal and Vertical Lines
Equation of a Line: Two Point FormLet's say we have a line passing through two point A(\(x_1\),\(y_1\)) and B(\(x_2\),\(y_2\)).
Let's take a point C (x,y) on the line and between A and B as shown below.
Attachment:
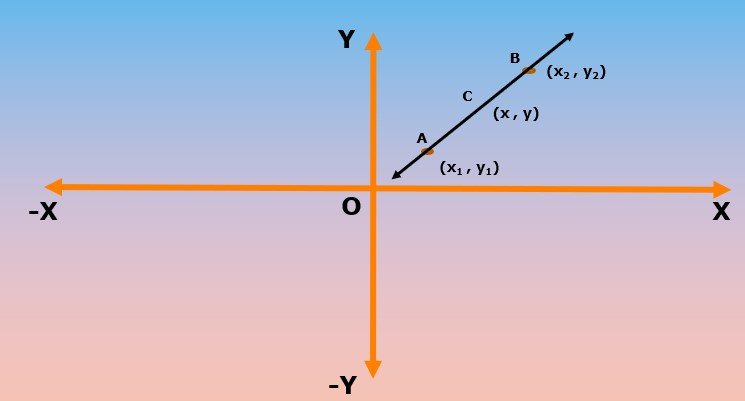 EL img-1.jpg [ 20.58 KiB | Viewed 651 times ]
EL img-1.jpg [ 20.58 KiB | Viewed 651 times ]
Slope of the Part CA of the line = Slope of the part BA of the line = Slope of the line
=> \(\frac{y - y_1}{x - x_1}\) = \(\frac{y_2 - y_1}{x_2 - x_1}\) [
Watch this video if you want to know about the slope of the line ]
=>
Equation of a line in Two Point Form as y - \(y_1\) = \(\frac{y_2 - y_1}{x_2 - x_1}\) * (x - \(x_1\))
where x and y are variables and value of (\(x_1\),\(y_1\)) and (\(x_2\),\(y_2\)) will be given to us in the problem.
Equation of a Line: Point and Slope FormLet's take the same line which was passing through point A and B and has a slope m
Attachment:
 EL img-1.jpg [ 20.58 KiB | Viewed 651 times ]
EL img-1.jpg [ 20.58 KiB | Viewed 651 times ]
Slope, m = \(\frac{y_2 - y_1}{x_2 - x_1}\)
Using, y - \(y_1\) = \(\frac{y_2 - y_1}{x_2 - x_1}\) * (x - \(x_1\))
Equation of a line in Point and Slope Form as y - \(y_1\) = m * (x - \(x_1\))
Equation of a Line: Intercept FormLet's say we have a line which intercepts X-Axis at point A(a,0) and Y-Axis at point B(0,b), as shown below.
Attachment:
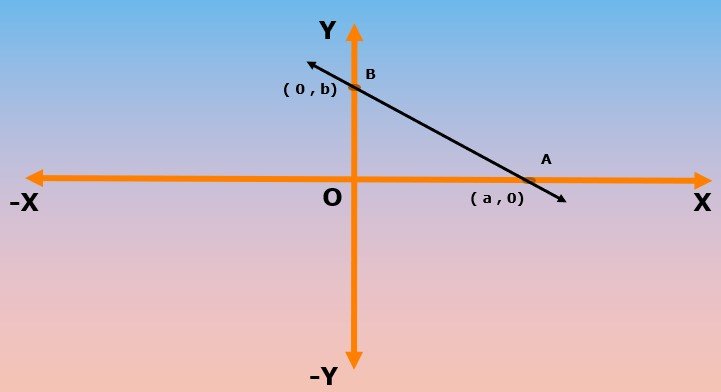 EL img-2.jpg [ 20.17 KiB | Viewed 643 times ]
EL img-2.jpg [ 20.17 KiB | Viewed 643 times ]
Using, \(\frac{y - y_1}{x - x_1}\) = \(\frac{y_2 - y_1}{x_2 - x_1}\) we get
\(\frac{y - 0}{x - a}\) = \(\frac{b - 0}{0 - a}\)
=> ay = -bx + ab
=> bx + ay = ab
Dividing both the sides by ab we get
Equation of a line in Intercept Form as \(\frac{x}{a}\) + \(\frac{y}{b}\) = 1
Generic Equation of a line (Point and Intercept Form)Let's say we have a line which intercepts Y-Axis at point B(0,b) and has a slope m, as shown below.
Attachment:
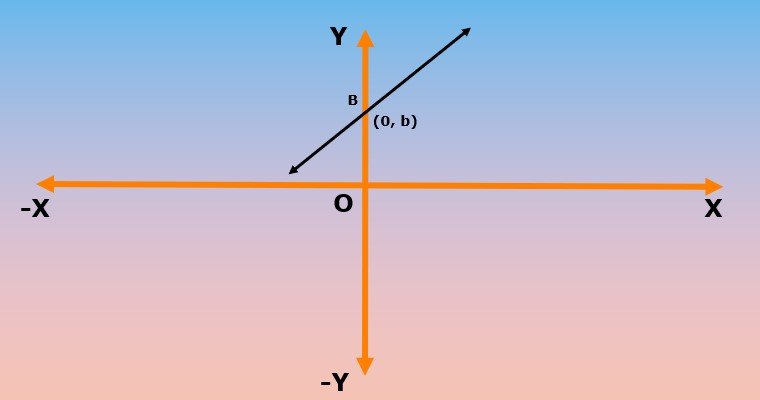 EL - generic equation.jpg [ 20.28 KiB | Viewed 659 times ]
EL - generic equation.jpg [ 20.28 KiB | Viewed 659 times ]
Using, y - \(y_1\) = m * (x - \(x_1\)) and substituting the value of Point B we get
y - b = m * (x - 0)
Generic Equation of a line (Point and Intercept Form) as y = mx + b
where m is the slope of the line and B is the y intercept.
Equation of horizontal and vertical linesLet's say we have a line parallel to X-Axis and intersecting Y-Axis at point B(0,b) and
a line which is parallel to Y-Axis and intercepting the X-Axis at point A(a,0) as shown below
Attachment:
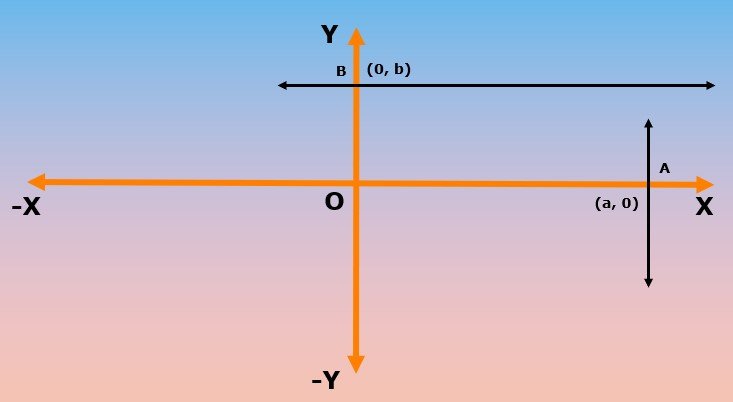 EL - Horizontal and parallel lines.jpg [ 21.02 KiB | Viewed 638 times ]
EL - Horizontal and parallel lines.jpg [ 21.02 KiB | Viewed 638 times ]
Equation of Horizontal LineNow, all the points on this line will be at the same distance b from X-Axis and will have the y-coordinate as b
=>
Equation of Horizontal line will be y = b [constant]
Equation of Vertical LineNow, all the points on this line will be at the same distance a from Y-Axis and will have the x-coordinate as a
=>
Equation of Vertical line will be x = a [constant]
Watch the following video to learn the Basics of Co-ordinate GeometryHope it helps!
Good Luck!
_________________

