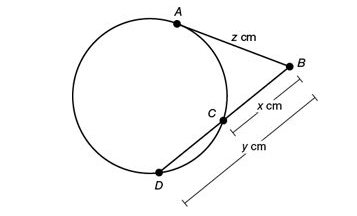
In the figure above, AB, which has length z cm, is tangent to the

dave13 , cosmopolitanism meets metaphor meets smiley face ... Classic.

This question is not about area or circumference.
It involves the "power of a point" theorem, one version of which is called the "secant-tangent theorem."
I'll put reference material below.

BUT: you do not need to use the secant-tangent theorem.

I will use the theorem in Method II because a harder question would not give us \(z=\sqrt{xy}\)
The power of a point theorem has three iterations, is very useful, and is not hard.
Initially it might appear so. Read material linked below.

We do not need the theorem because this part is given: \(z=\sqrt{xy}\)
The variables are lengths, thus positive. Square both sides: \(z^2=xy\)
See below. That equation is the essence of the secant-tangent theorem.

I. WITHOUT the theorem - Unique value for \(x\)?

(1) \(CD = x\) cm
\(y - CD = x\)
\(y - x = x\)
\(y=2x\)

Given: \(z=\sqrt{xy}\)
\(z=\sqrt{x*2x}=\sqrt{2x^2}=x\sqrt{2}\)
\(z=x\sqrt{2}\)
Two variables, one equation. Insufficient.
OR: we know nothing about \(z\), hence nothing about \(x\)

Or: test numbers that make this statement true: \(z=x\sqrt{2}\)
If you can get two different values for \(x\), #1 is insufficient
Let \(x = 25\) and \(z = 25\sqrt{2}\)
Let \(x = 2\) and \(z = 2\sqrt{2}\)
Two values of x = not unique
INSUFFICIENT alone. Cross off A and D

(2) \(z = 5\sqrt{2}\)
\(z=\sqrt{xy}\)
\(z = 5\sqrt{2}\)
\(5\sqrt{2}=\sqrt{xy}\)
Two variables, one equation. Insufficient.
OR: we know nothing about \(y\), hence nothing about \(x\)

OR test numbers.
\(z=\sqrt{xy}\)
\((5\sqrt{2})=\sqrt{xy}\)
Let \(x = 5\) and \(y = 10\)
Let \(x = 10\) and \(y = 5\)
#2 does not yield a unique solution for \(x\). Insufficient. Cross off B.

C or E?
Combine #1 and #2:
#1, expanded:
\(y = 2x\)
\(z=\sqrt{xy}\)
\(z=\sqrt{x*2x}=\sqrt{2x^2}\)

Combined #1 and #2
\(5\sqrt{2}=\sqrt{2x^2}\)
One variable, one equation. Sufficient. Or solve
\(5\sqrt{2}=x\sqrt{2}\)
\(x=5\) That works

Answer C

II. Secant-tangent theorem

In the diagram, point B lies outside the circle.
AB (= z) is tangent to the circle
BD is a secant, a line that intersects a circle at two points
BD (= y) has two segments, BC (= x) and CD

The secant-tangent theorem states
\((AB)(AB)=(BC)(BD)\)
\((AB)^2=(BC)(BD)\)
In this case, using the theorem with given lengths, we have
\(z^2=xy\)

We need information about \(y\) and \(z\) to get a unique value for \(x\)

(1) \(CD = x\)
Inspect the diagram: \(y=2x\), or

\(y - CD = x\)
\(y - x = x\)
\(y=2x\)
From secant-tangent theorem:
\(z^2=xy\)
\(z^2=(x*2x)=2x^2\)
Two variables, one equation.
Insufficient.

Test numbers:
Let \(x = 1\) and \(z = \sqrt{2}\)
Let \(x = 2\) and \(z = \sqrt{8}\)

Or, we know nothing about value of \(z\), so we still know nothing about \(x\).
#1 alone is insufficient. Cross off A and D

(2) \(z = 5 \sqrt{2}\)
From the secant-tangent theorem, we know \(z^2=xy\)
\((5\sqrt{2})^2=xy\)
\(50=xy\)
Two variables, one equation. Insufficient.

OR, we know nothing about \(y\) and hence nothing about \(x\)

OR test numbers.
\(50=xy\)
Values: \(x=2\) and \(y=25\)
\(x=10\) and \(y=5\)
\(x\) does not have one unique value - insufficient

(2) alone is insufficient. Cross off B.

(C) or (E)?
Combine #1 and #2
\(z^2=2x^2\) and \(z=5\sqrt{2}\), so
\((5\sqrt{2})^2=2x^2\)
One variable, one equation: sufficient
If you need to, do the math:
\((5\sqrt{2})^2=2x^2\)
\(50=2x^2\) --> \(x=\sqrt{25}\) --> \(x=5\) (length cannot be (-5)

Answer C

Hope that helps.

Good reference material:
A simple overview and ways to remember each power theorem is HERE
Secant-tangent with a simple proof is HERE.
Rules for Chords, Secants, and Tangents in a Circle are HERE.
Power of a Point Description and Problems are HERE
Power of a Point Theorem - material is short and dense

Then see the the page linked above, at the Power of a Point section.

P.S. mikedays , I answered your query via PM.
_________________

Responding to a pm:
The tangent-secant theorem tells us that Tangent^2 = Whole Secant * External Secant
So whenever the length of 2 of these is defined, the third is automatically fixed.

Here,
AB = z,
BC = x
BD = y

\(z^2 = y*x\) (also given in the question so that you don't need to remember the theorem)

1. CD = x cm
y = 2x
We have no numerical value for any length, x, y or z. There is no way we can get the numerical value of x from this information.
Not sufficient.

2. \(z = 5\sqrt{2}\)
\(5\sqrt{2} = \sqrt{xy}\)
xy = 50
x can be 5, y can be 10
If we move BD slightly up, x will be less than 5 and y will be more than 10 (but the product will stay 50)

Value of x is not unique. Not sufficient.

Using both statements,
x*2x = 50
x = 5
Sufficient.

Answer (C)
_________________

Sol- we are given and also by second theorem that z^2=xy
1- this gives y=2x--> z^2=2x^2
2-\(z=5\sqrt{2}\)...not given x or y

Combining we know
\(5\sqrt{2}^2=2x^2\)

From 1 statement we know y = 2x
And then z = x√3 but to calculate z we need it's value
From 2 statement we know will get z
So it's together sufficient

study mode

carcass , Can you please let us know . How this question can be solved.

Thanks.

Given: z = Root(X*Y) -> to answer question we need to have information about z and y

1) y = 2x -> z=Root(x*2x) = Root (2x^2) = x*root(2) -> however INSUFF. as we have no information about x
2) z=5*root(2) clearly Insuff as we don't have any details about x or y

However combining (1) + (2) you get x*root(2) = 5*root(2) => x=5 , therefor SUFFICIENT

Hi

You can go through the solutions posted in this thread.
You can also read about circles theory here:

https://gmatclub.com/forum/math-circles-87957.html

Bunuel, chetan2u could you please share your approach here? Thanks.

In the OG2018 explanation of this answer it is said that only by looking at statement 2) one can conclude that CD is a diameter of the circle. How do we get to that conclusion?

hey generis today is international day of indepencence so i tried to solve the above DS question independently, i ve read whole thread also visited this link...

but i smply couldnt understand how to approach the problem...

i know formula of area of circle PiR^2 and also circumference 2PiR

i know what is tangent, what is diametr and radius, but still couldnt wrap my mind aroud this problem what rule to apply here , can you help please

have a fantastic day

generis many thanks for great explanation... just in the end the last part about math it was like "tail wagging the dog "
you know i think the root of my gmat quant problem, is in root

If you need to, do the math. (i would love to do the math)

\((5\sqrt{2})^2=2x^2\) why after you sqauare both sides

\(50=2x^2\) --> the 2x^2 remains intact?

OR why didnt you write initially

\((5\sqrt{2})^2=(2x^2)^2\) you squared RHS, why didnt you apply the same pattern to \(2x^2\)

dave13 - too much staring at numbers?

I would bet you missed the difference between \(z^2\) and \(z\)

The equation is: \(z^2=2x^2\)

\(z\) by itself is: \(z=5\sqrt{2}\) -- Not squared yet. Plug \(z\) INTO the \(z^2\) equation

\(z^2=2x^2\)
\((z)*(z) =2x^2\) Leave RHS alone. That's what \(z\)-squared equals
\(z=5\sqrt{2}\)
\((z)*(z) =2x^2\)
\((5\sqrt{2})*(5\sqrt{2})=2x^2\)
\(5*5*\sqrt{2}*\sqrt{2}=2x^2\)
\(25*2=2x^2\)
\(50=2x^2\)
\(\frac{50}{2}=x^2\)
\(25=x^2\). Take the square root of both sides:

\(x=5\) (lengths cannot be negative)

I hope that helps.
_________________

Hi generis,

Thanks for your explanation. Could you copy here your explanation to mikedays' query?

Thanks!!

Would someone kindly look at the diagram from the solution given by OG?

Based on ONLY the 2nd data point (2): z = 5√2

How are we able to determine that CD is the diameter of the circle?
And that the triangle AOB is a right-angle triangle?

Hi lassakul

Sometimes the explanations in the OG are just complex and not easy to follow or comprehend.

I would advise you to look at it from the power of point theorem as explained in the above posts.

In the figure above, AB, which has length z cm, is tangent to the circle at point A, and BD, which has length y cm, intersects the circle at point C. If BC = x cm and \(z = \sqrt{xy}\), what is the value of x ?

(1) CD = x cm

This mean y = 2x

INSUFFICIENT.

(2) \(z = 5 \sqrt{2}\)

INSUFFICIENT.

(1&2)
\(z = \sqrt{xy} = 5 \sqrt{2}\)

\(\sqrt{xy} = \sqrt{2x^2} = \sqrt{2}x\)

\(x = 5\)

SUFFICIENT.

Answer is C.
_________________

In the figure above, AB, which has length z cm, is tangent to the circle at point A, and BD, which has length y cm, intersects the circle at point C. If BC = x cm and z=√xy, what is the value of x ?

(1) CD = x cm
This tells us : z=√2x^2
Insufficient.

(2) z=5√2
Clearly insufficient

C: Sufficient.
_________________

Solution:

We need to determine the value of x.

Statement One Alone:

Notice that the combined length of CD and BC equals the length of BD; thus, y = 2x. Substituting this into the equation z = √(xy), we obtain:

z = √(xy) = √(x * 2x) = √(2x^2) = x√2

Since we know nothing about the value of z, we cannot determine the value of x. Statement one alone is not sufficient.

Statement Two Alone:

We know z = 5√2 and we know z = √(xy); however, without a relation between x and y, this is not enough information to calculate the value of x. For instance, if y = 25 and x = 2; then √(xy) = √(50) = 5√2. In this case, the value of x is 2. On the other hand, if y = 10 and x = 5, then again √(xy) = 5√2, but in this case, the value of x is 5.

Statement two alone is not sufficient.

Statements One and Two Together:

Using the analysis of statement one, we know z = x√2 and using statement two, we know z = 5√2. Setting the two expressions of z equal to each other, we obtain x√2 = 5√2, which implies x = 2. Statements one and two together are sufficient.

Answer: C
_________________

Hello from the GMAT Club BumpBot!

Thanks to another GMAT Club member, I have just discovered this valuable topic, yet it had no discussion for over a year. I am now bumping it up - doing my job. I think you may find it valuable (esp those replies with Kudos).

Want to see all other topics I dig out? Follow me (click follow button on profile). You will receive a summary of all topics I bump in your profile area as well as via email.
_________________