S97-14

Official Solution:

A circle is inscribed in an equilateral triangle, such that the two figures touch at exactly 3 points, one on each side of the triangle. Which of the following is closest to the percent of the area of the triangle that lies within the circle?

A. 50%
B. 55%
C. 60%
D. 65%
E. 70%


First, draw a picture of the circle and triangle and make up good labels for all points.



We should add one more point: the center of the circle, which will also be the center of the triangle. Let's call that point O.

In order to deal with the area of the circle, we'll need a radius (with circles, it should always be your first instinct to draw in a radius). We'll put in OZ.



Now, because both equilateral triangles and circles are regular, symmetrical figures, and because they're lined up perfectly in this scenario, we can take advantage of "symmetry arguments." For instance, it should be obvious that each of the points X, Y, and Z are the midpoints of their respective sides. You don't need to prove this, nor do you want to under time constraints. Rather, on the exam you should look for regular, symmetrical figures to behave symmetrically, and points that seem like midpoints can actually be proven to be just that. Likewise, we can draw in AO, and we know that this line cuts angle BAC perfectly in half. If you've drawn the figure carefully, this conclusion should be apparent. (We almost have the Deathly Hallows here, for you Harry Potter fans).



Now, since any angles in an equilateral triangle are \(60^\circ\), \(\angle OAZ = \frac{60^\circ}{2} = 30^\circ\). Moreover, angle AZO is \(90^\circ\) (a radius that touches a tangent line will form a right angle).

Thus, we know AOZ is \(60^\circ\), and we have a 30-60-90 triangle. Let's redraw that triangle by itself:



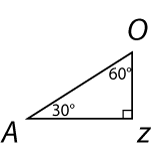
Now, let's make up a convenient radius. Say \(OZ = 1\). We quickly get the dimensions of triangle AOZ: 



We can now compute the areas of each figure. First, the circle is \(\pi r^2 = \pi(1)^2 = \pi\).



The triangle is a little harder, but we're almost there. Since \(AZ = \sqrt{3}\), and Z is the midpoint of AC,  we know that \(AC = 2 \sqrt{3}\).



If you know the formula for the area of an equilateral triangle in terms of its side length, you can plug in right now -- or you can figure out the height. Let's take the latter approach. 

Since we know \(AO = 2\), and \(OY = 1\), we know that \(AY = 3\) (again, we don't need or want a rigorous proof that they form a straight line. Regular figures behave nicely!)



And again by symmetry, this tells us that \(BZ = 3\) as well:

Now we can calculate the area of the triangle, which equals \(\frac{1}{2}bh = (\frac{1}{2})(2 \sqrt{3})(3) = 3 \sqrt{3}\). (Incidentally, the area of an equilateral triangle in terms of its side length \(s\) is \(\frac{s^2 \sqrt{3}}{4}\), which in this case gives \(\frac{(2 \sqrt{3})^2 \sqrt{3}}{4} = \frac{12 \sqrt{3}}{4} = 3 \sqrt{3}\))

Finally, we compute the ratio of the areas: 

\(\frac{\text{Area of circle}}{\text{Area of triangle}} = \frac{\pi}{3 \sqrt{3}}\). We approximate:  \(\pi \approx 3.1\) and \(\sqrt{3} \approx 1.7\), so we get \(\frac{3.1}{3(1.7)} = \frac{3.1}{5.1} \approx \frac{3}{5} = 60%\).

(Excel gives the ratio as 60.46% to a couple more decimal places, so our approximation didn't create much of an error).

If you get completely stuck on this problem, of course learn the method above. But a scrappy method that's really not too bad is to draw the picture carefully and try to eyeball the answer. Since the answers only differ by 5%, this might only help you eliminate extremes, but that's better than nothing. And incidentally, if you must guess at random on such a problem, in which you have to estimate a ratio of areas or lengths that you can draw reasonably approximately, guess toward the middle of the pack. The question writers will have a sequential lineup of answers, and it's unlikely that the writers would put all the wrong answers on one side of the right answer, because you would be able to knock out the extreme wrong answer or answers more easily. In this case, you'd be unlikely to see the answers as (A) 60% (B) 65% (C) 70% (D) 75% (E) 80% or as (A) 40% (B) 45% (C) 50% (D) 55% (E) 60%.


Answer: C