2 Clues that you are missing on Data Sufficiency

Data Sufficiency really is a game unto itself. At Veritas Prep, we like to tell our students to "Think Like the Testmaker"… Why are they writing things a certain way; why did they add a particular word; why did they phrase it that way? Sometimes, the GMAT Testmakers will set traps for you, but other times, they are just leaving clues. If you can train yourself to recognize these clues and leverage them, you can be much more efficient in both your problem-solving abilities and your time management on this challenging exam.

This is especially true for Data Sufficiency problems and there are two clues you probably are not using nearly as often as you should: the "Combination Question", and the "Obvious Statement." In this article, we're going to break each of these clues down for you, so you can be prepared come test day.

The Combination Question

This is one of our all-time favorite question types. Anytime the GMAT asks you for a "combination" of variables, that should be a clue to you that you'll want to get to work solving directly for that combination instead of looking for individual parts of the question and then combining them later, which you may be tempted to do.

Let's look at a sample problem:

The first thing you should notice when looking at this problem is that it is asking you for a combination, "the sum" of the two perimeters of the triangles. Remember, if the Testmakers are asking you for a combination of items, they will almost always make it easier to solve the question with less information, so we should be trying to see how we can answer this question with just the information presented in Statement 1 (or Statement 2).

When initially tackling this problem, most people think the answer is C - that they need both statements to answer the question. This is because they look at Statement 1 and think, "Well I know that AE is 25, but I'm not sure how that is broken up between AC and CE. If I don't know how long those sides are, I cannot find the perimeter of each triangle and the sum of those perimeters. I need Statement 2 to find the lengths of those sides." But remember, we don't want to find the individual perimeters of these triangles - we want to go straight to the combination.

A great way to do this in this particular problem is to start by labeling the diagram. This will help us determine what we actually need in order to solve the problem. Here, we've labeled our diagram with "x" and "y" to represent the lengths of each side. We know both triangles are equilaterals, so we know that the sides of each respective triangle are equal.



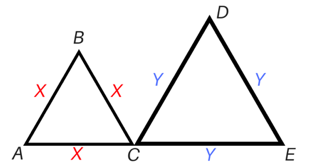
So, what do we need to find the combination of both triangle perimeters? We need 3x + 3y. Looking at the statements again, Statement 1 tells us the combinations of one of those sides: x + y is equal to 25. If we triple that, we will get 3x + 3y to find the combination of perimeters. Therefore, the correct answer is A.

Even though well more than half of people get this wrong, notice that the math isn't incredibly hard on this problem. What's difficult is the way you tend to do math - we've learned to "solve for x", so if we are asked for a combination of x and y, we assume we need to solve for x, solve for y, and then combine those two together. In reality, when a Data Sufficiency question asks you about a combination of variables, you can (almost) always solve for that combination with less information than you'd need to solve for the variables first and then combine. So pay attention when you're asked about a sum or a difference as opposed to a single variable - that's your cue that the right answer is probably "surprisingly sufficient" so you should roll up your sleeves and get to work accordingly.

The Obvious Statement

Anytime one statement is completely obvious on a Data Sufficiency problem (For example, there's NO WAY someone could be answer "B" on this question!), you should be wary of automatically thinking that the problem has just become easier.

On questions like this, you should be asking yourself, "Why would the Testmakers throw away a statement like that?" The answer is either that they are trying to lay a trap for people who are not thinking critically, or is that there is a clue they know people can leverage if they look at the problem correctly.

Take a look at the following example question:


Looking at this question, straight away, Statement 2 should jump out at you as being pretty obvious - no one in their right mind would say that "y is not a prime number" is sufficient by itself to finding the value of integer z. But remember, when one statement is obvious, it's either a trap or it's a clue. Either way, it tells you something you should be really thinking about when evaluating the other statement.

Very often, people pick A for this question. This is because when they take a look at the first statement, they start by picking simple numbers to plug in place of "y". Let's try, for example, y = 5 and y - 1 = 4. In this case, when y is divided by "y - 1", the remainder will be 1 (5/4 = 1 with a remainder of 1). Using this strategy, you will see that almost any integer (notice how we say almost here), when used in place of y, will give you a remainder of positive 1 when divided by "y - 1." 10 divided by 9, 200 divided by 199, 99 divided by 98 - they all give you 1 remainder 1. This logic seems to make sense, right? Now, like most test-takers, you can just choose answer choice A and move on.

Not so fast! Now that you know how to "Think Like the Testmaker", you can figure out how to avoid this trap answer. As we mentioned before, Statement 2 should stick out to you as an obvious clue - why would "prime" vs. "not prime" matter here? Well, let's think about the lowest prime number (and the only even prime number), 2. Would 2 work in place of "y" here? If y = 2, then y - 1 = 1. 2/1 is 1 with a remainder of 0, which is a different outcome than when we tried plugging in numbers before. Now we see that, unless we have Statement 2 telling us that y is not prime, we really cannot be sure what remainder we will get when y is divided by y - 1. You need both statements to solve the problem, so our answer is actually C. (Note that the other chance you have to "save yourself" from trap answer A here is to always consider the lowest and highest values they'll let you use: if you saw that the lowest pair of consecutive positive integers is 2 and 1, you may not need the clue from statement 2 to find that option of 2/1 = 0 with no remainder. Either way, recognize that well more than half of people pick A so it is important to pick up on clues and to use good strategy so that you can avoid these tempting wrong answers!)

Remember, when you see a dead obvious statement in a Data Sufficiency problem, it's either a trap answer or it is a clue; either way, there is a reason it is there so make sure you spend some time trying to figure out why.

Alright, let's summarize those two clues we just discussed. On Data Sufficiency questions, make sure you're looking out for:

1) Combination Questions
2) Obvious Statements

By leveraging these two clues, you'll be able to save yourself from making mistakes that could otherwise be easily avoided, and better determine how to spend your time on these challenging Data Sufficiency questions.

This article was written by Brian Galvin, Chief Academic Officer at Veritas Prep and aficionado of the board game Clue. Do you have questions about how Veritas Prep can help you improve your Data Sufficiency skills and achieve your highest possible GMAT score? Learn more about their various services and free resources here.