Hi,
To find the equation of the reflected line just keep in mind the following things:--
1. Each and every point on the reflected line has the same distance from the line on which the reflection happened as the corresponding point on the original line.
2. The line on which reflection happens is the perpendicular bisector of the line joining a point on the original line and the corresponding point on the reflected line.
So, to find the equation of the reflected line you need to find two points.
One point you can get by finding the point of intersection of the original line and the line on which the reflection happened.
To find the other point you can take any point on the original line and drop a perpendicular to the line on which reflection has to happen. And find the point of the intersection of the line on which the reflection has to happen and the perpendicular drawn just now.
Now, the point of intersection which we got just now is the mid point of the point on the original line and the point on the reflected line. So, we can find the point on the reflected line too.
Will give an example : ( Figure attached )
Suppose we are given the equation of line l and we have to find the reflection of l on line m
line n is the reflected line whose equation we need to find.
We can find one point (O) by solving for lines l and m
Now to find one more point on line n (C(x2,y2)):
Take a point A(x,y) on line l and draw a perpendicular to line m (point B)
Now , we know the slope of line m so we can find the slope of any line perpendicular to line m and we have one point (A) through which that perpendicular is passing so we can find equation of that perpendicular and hence we can find point B (x1, y1)
Since, B is the midpoint of AC (since the line on which reflection happens is the perpendicular bisector of any two corresponding lines on the original and the reflected line)
So,
x1 = (x+ x2) /2
and
y1 = (y + y2)/2
So, we can find x2,y2 and hence point C
Once we have point C then we can find equation of line n as we have two point O and C
Now coming to your doubt:
What if -
1. A line with the equation y = px + q is reflected over the line y = 2x, How shall we approach this question?
2. A line with the equation y = px + q is reflected over the line y = 5, How shall we approach this question?
For the 1st one i will go with the approach mentioned above.
For the second one since the line is y=5. So the X co-ordinate of the corresponding point on the reflected line will remain same but for finding the y co-ordinate i will follow the procedure mentioned above.
Hope it helps!
Attachments
File comment: File attached
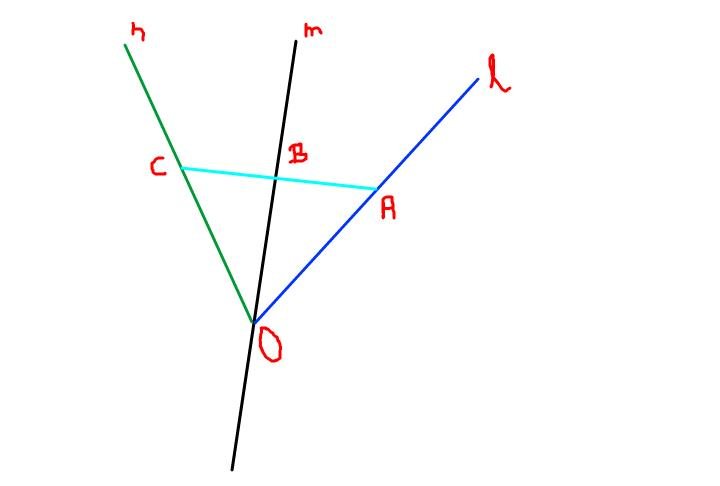
image.JPG [ 14.48 KiB | Viewed 14131 times ]

 95%
(hard)
95%
(hard)
 72%
(01:51)
wrong
72%
(01:51)
wrong  based on 183
sessions
based on 183
sessions
