A small School has three foreign language classes,one in French,one in

Hi Mike ,

Shouldnot 34 includes student who learn Spanish only , spanish and french , and spanish and german .

So why you did not include Spanish and German .

Dear Ganganshu,

I'm happy to respond.

My friend, in GMAT math, it's extremely important to distinguish what is required by mathematical rules and what is strategic. For example, if we have to solve the very simple equation 3x + 7 = 49, there would be nothing mathematically wrong to begin by multiplying both sides by 13, but that would be a spectacularly poor move in turns of strategy. That's a very simple obvious example, but you always have to be thinking in terms of both what is mathematically true and what can we do for strategic purposes.

In the prompt, the 34 students in French absolutely includes
(a) the French only students
(b) the French & Spanish students
(c) the French & German students
(d) any students in all three language classes.
That's big-picture true. In the overall picture of what is happening in this problem, this undeniably true.

Then, we get to Statement #1, which gives us a ton of information about Spanish and Spanish-French, but doesn't mention German even once. This suggests an extremely valuable strategy: let's ignore German. Let's simply pretend, for the sake of analyzing this particular statement, that the German class doesn't exist. Admittedly, this is not "true" in the largest view of the problem, but it's a simplifying assumption that allows us to focus on one aspect of problem-solving, and as it happens, the simplifying assumption leads to a definitive answer to the prompt question.

Compare this to another type of problem. Suppose I had to solve the inequality \(x^2 +4x - 45 > 0\). There are several methods of solution, but one would be to begin by solving the equation: \(x^2 +4x - 45 = 0\). It's perfectly true that in doing so, we are solving something different from what the question asked, and it's also true that the values that satisfy the equation absolutely will not satisfy this inequality. Nevertheless, this is highly productive from a strategic point of view, because the solutions to the equation form the boundary conditions that will allow us to "chunk" the number line and figure out the regions that work and don't work. The solutions to the equation are x = -9 and x = +5, and the solution to the inequality are x < -9 and x > +5.

Sometimes it is a highly strategic move to assume that something that is slightly different from what is true in the problem scenario in order to reach a solution. This happens in many areas of GMAT math, and the inability to see these routes of solution can be a huge deficit on the GMAT Quant.

Does all this make sense?
Mike

Hey mike,

What if B = 11, F = 16, S = 23 which satisfies both the conditions that S=34(23+11) and F = 27(16+11) and S+F+B = 49(23+16+11).
However the answer to the Question asked becomes 11.

If you consider B=12, F=15, S = 23 which again satisfies all the above conditions, the answer to the question asked becomes 12.

So, i m not sure how A is sufficient to answer the Question ? I m not sure if my reasoning is right or if I'm missing something.

thanks,
Nitish

Hi nitishms! Carolyn from Magoosh here - I'll step in for Mike

It looks like you may have just made a few small calculation errors here! In the first case, you said S+F+B = 49(23+16+11). But when we add 23+16+11, we get 50 (not 49). So this case doesn't work.

And looking at your second case, if B = 12, F = 15, and S = 23, then the total number of people taking Spanish will be 23+12 = 35, not 34. So this case also doesn't work.

We can definitively solve this using the two equations that we can determine:

S + 2B + F = 61
S + F + B = 49

This system only has one solution for B, which is 12.

I hope that helps clear things up!
-Carolyn

Hi Mike

Thank You, you actually made it easy for us.

Though I need your help, my approach to this question was like any other I would have used when 3 Venn diagrams are involved.

With 3 Vds, am unable to identify the value of "d" (attachment) to get my answer. I had done my rough which was not great to share , hence just sharing the diagram I had made and followed.

Please can you review this and advice, what am I missing.

Regards
Abhinav

Hello Abhinav,

Mike has already explained why it is in our strategic interest to leave German aside in order to be able to solve this question. The reason that was stated for doing so is that German is never mentioned in Statement 1.

However, I do not know how to convince myself or you that it is the best way to approach a 3 Venn diagram problem in GMAT. I do not know if we can call this a thumb rule : "if one of the 3 groups isn't mentioned in a statement, pretend it doesn't exist".

The only reason that explains why somehow this approach, though seemingly absurd at the first glance, finds consensus among pretty much everyone in this thread is that it is a retired GMAT problem and the OG seems to have followed the same approach in its explanation, though it didn't explain why.

So I will urge anyone to please answer this: is this a general rule/strategy that we can follow?

We are trying to solve for how many of the Spanish Students also take French. We can set up a Venn Diagram as such (figure 1)
We know that A + B = 34 (total number of people who are taking Spanish), we are trying to solve for B

STATEMENT 1:
We know that there are a total of 27 french students (B + C = 27) and that 49 students enrolled in either French Class, Spanish Class or Both (A + B + C = 49)
We have 3 equations and 3 variables, so we can solve for B!
Statement 1 is sufficient

STATEMENT 2:
It tells us that 1/2 of the 34 Spanish Students are enrolled in either German or French. Clearly it's not enough information to get the exact # of students who are taking french, as we don't know the total # of French students, # of German students and # of overlap between all of the groups
Statement 2 is insufficient!

The answer is A

Answer: Option A

Video solution by GMATinsight



Get TOPICWISE: Concept Videos | Practice Qns 100+ | Official Qns 50+ | 100% Video solution CLICK HERE.

Two MUST join YouTube channels for GMAT aspirant GMATinsight (1000+ FREE Videos) and GMATclub
For a Comprehensive Topicwise Quant Video course at an affordable price CLICK HERE

Hi, avigutman BrentGMATPrepNow, CrackVerbal, can you please help us understand what all regions 49 includes?

My understanding is that 49 includes only F + Only S + Both F&S + Both F&G + Both S&G + All three. In the context of the question, 49 should also include Both F&G and S&G because that way we do get either French or Spanish.

Thank you!

Let's analyze the question here.

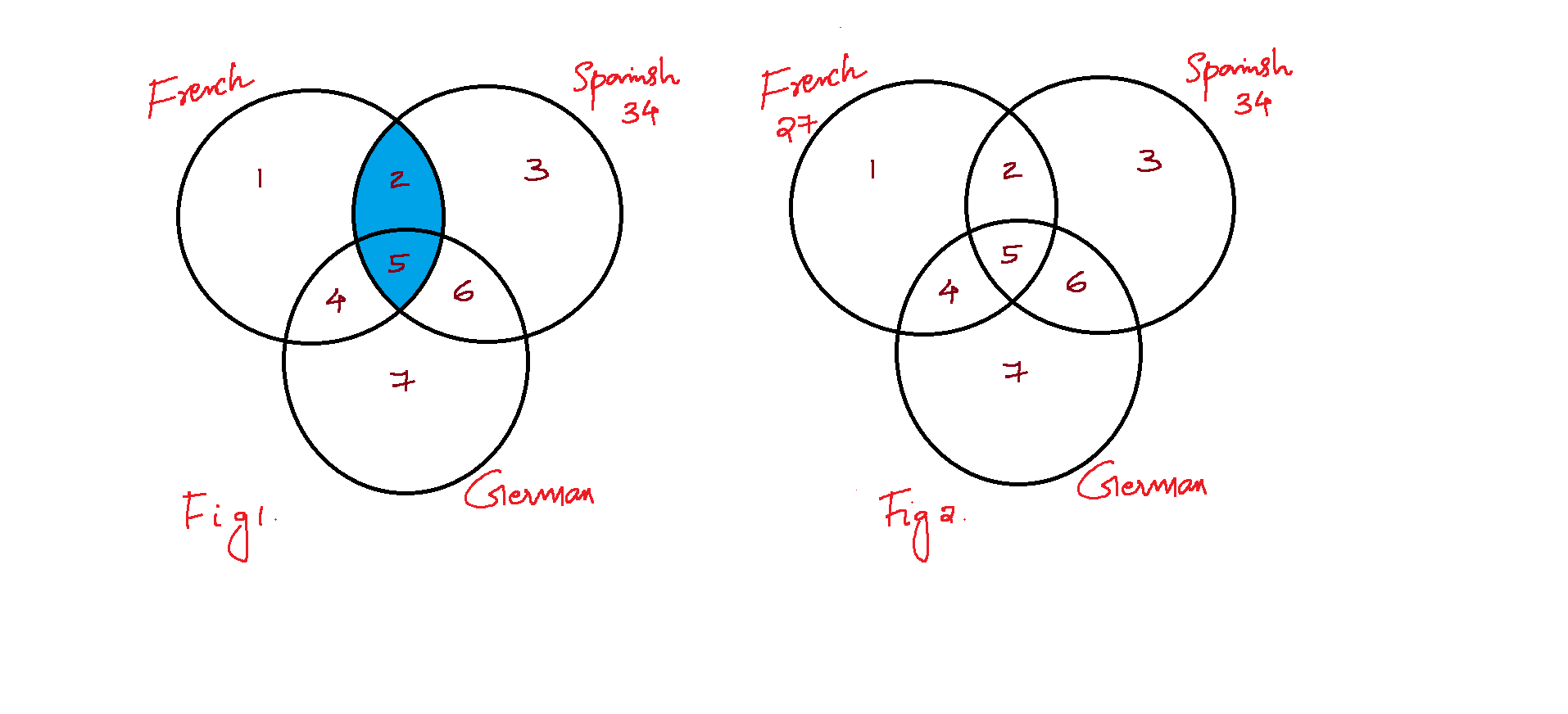
A small School has three foreign language classes, one in French, one in Spanish, and one in German. How many of the 34 students enrolled in the Spanish class are also enrolled in the French class?



Before moving to the explanation, how we define each region in the Venn diagram is very important.

Region 1 -Only French, 3 -Only Spanish, 7 - Only German

Region 2- Only French and Spanish, 4 - Only French and German, 6 - Only Spanish and German

Region 5 - All three - Spanish, French, and German

The number of the students enrolled in Spanish = n(Spanish ) = Region 3 + 2 + 5 + 6
The number of the students enrolled in French = n(French ) = Region 1 + 2 + 4 + 5
The number of students enrolled in both Spanish and French = Region 2 + 5
The number of students enrolled for both Spanish and German = Region 6 + 5

The number of students enrolled for both French and German = Region 4 + 5

The number of students enrolled in Spanish classes is 34 i.e n(Spanish) = 34 and we are asked to find the number of students enrolled in both Spanish and French classes i.e n ( both french and Spanish) which is represented by Region 2 + 5 in figure 1 attached.

(1)There are 27 students enrolled in the French class, and 49 students enrolled in either the French class, the Spanish class, or both of these classes.

Refer to fig 2 in the picture attached.

From St 1, it's given that n(French) = 27 and n(French U Spanish ) = 49 = Region 1 + 2 + 3 + 4 + 5 +6
We already know that n(Spanish) = 34.
n(French U Spanish ) = n(French) + n(Spanish) - n ( both french and Spanish)

49 = 27 + 34 - n ( both french and Spanish)

n ( both french and Spanish) = 61 - 49 =12.

Since we get a definite answer for the Q.stem, Statement 1 alone is sufficient to answer.

(2) One-half of the students enrolled in the Spanish class are enrolled in more than one foreign language class.

We know that n(Spanish) = 34 and half of them i.e 17 enrolled in more than one foreign language class.

17 = Region 2 + 5 + 6

We are asked to find Region 2 + 5 in the Q.stem. Since we don't have any idea about region 6, Statement 2 alone is not sufficient.

I hope the explanation is clear to you and you got a clear idea about which region is represented by 49.

Thanks,
Clifin Francis,
GMAT Mentor.

KarishmaB avigutman I got my answer as 12 for Statement 1. But why are we ignoring German? The question should ask French and Spanish but not german. Then we can confidently say that 12 people take both French and Spanish

Hi Arsalan24, actually it's only thanks to the fact that the question stem didn't specify whether it's interested in German students that statement (1) is sufficient on its own. If we had to say how many of the 12 do or do not study German, we would have no way of making such an inference with statement (1) on its own.

Think about it in another way - what if the question told us that besides these 3 foreign languages, students study English, Math and Science too. And say the rest of the question was as it is. Would this new info make any difference to our solution? No. We are not bothered about who studies which other subjects etc.

Question: How many of the 34 students enrolled in the Spanish class are also enrolled in French class?
(We don't care how many of these are enrolled in German/English/Math.. etc)

(1)There are 27 students enrolled in the French class,and 49 students enrolled in either the French class,the Spanish class,or both of these classes.

We have all information about the French and the Spanish classes only. We are not given how many of these are in German or not in German. Since the question also asks about French and Spanish only and the statement also provides all relevant information about French and Spanish only, we can ignore German completely. It is just like English/Math etc - other classes that students are likely taking but we are not concerned about those.

Hi,

How to be always sure that our assumption is within the scope of the question. In this case, we could have easily chosen option E as we know 34 persons who enrolled for Spanish include people who enrolled for German as well and 27 persons who enrolled for French (as per statement 1) also include people who enrolled for German. This makes statement 1 insufficient and E should be the answer. Kindly clarify Bunuel KarishmaB BrentGMATPrepNow

Thank you for your help.

Number of people who enrolled in German is irrelevant to us as explained in my comment here: https://gmatclub.com/forum/a-small-scho ... l#p3054145
The question asks us only about Spanish and French.
Take a look and get back if you still have some doubts.

Hi everyone,

While I understand that "ignoring" german classes could be an extremely useful strategy in this question. I am not sure if this is something that can also be used for other questions. I am trying to check whether this strategy can be correlated to other such questions?
If anyone can share any links to similar OG questions of this type where using such a strategy has been helpful then it would be great for some practice.

Just want to be sure in identifying where using such a strategy would not be helpful.