What is the value of a + b/(ab)^1/2? (1) a is 4 times b.

You are not wrong - thank you for the correction!

An answer option will be sufficient if we have a unique answer.


\(a=4b\). Substitute these values, and you'll get
=>\(\frac{5b}{\sqrt{4a^2}}\)
=>\(\frac{5b}{2\sqrt{a^2}}\)
We know from the concepts of absolute values that the square root of any square of a variable is
\(\sqrt{a^2} = |a|\)
and \(|a|\) has three possible values - -a,0, and a.
Thus, for this reason we can conclude that the statement could give us more than one answer.
\(\frac{-5b}{2a}\), \(∞\), and \(\frac{5b}{2a}\)




This would give us the same scenario as statement (1).

(1)&(2) together, it'll still mean the same scenario.
There's no unique answer. E.

I think in GMAT sqrt(any number), always gives positive value. So i dont think E is the correct answer. It should be D.

Nope. I don't think so. Please check with the experts here to clarify!

a is a variable - It could a negative number or a positive.

\(\sqrt{a^2}\) can have three values -> -a, 0, and a.

\(\sqrt{a^2}=|a|\). The absolute value cannot be negative so \(\sqrt{a^2} \geq 0\).
When a < 0, then \(\sqrt{a^2}=|a|=-a=-negative=positive\).
When a > 0, then \(\sqrt{a^2}=|a|=a=positive\).
When a = 0, then \(\sqrt{a^2}=0=nonnegative\).

Generally, the square root function CANNOT produce negative result. EVER.

\(\sqrt{...}\) is the square root sign, a function (called the principal square root function), which cannot give negative result. So, this sign (\(\sqrt{...}\)) always means non-negative square root.


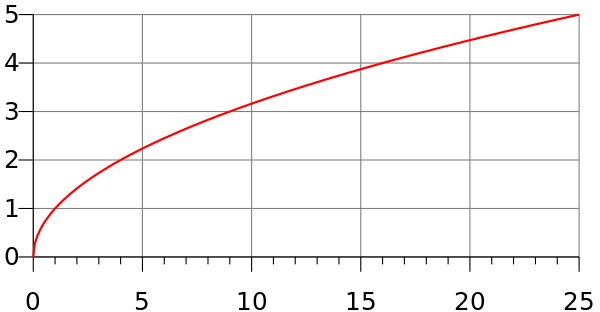The graph of the function f(x) = √x

Notice that it's defined for non-negative numbers and is producing non-negative results.

TO SUMMARIZE:
When the GMAT provides the square root sign for an even root, such as a square root, fourth root, etc. then the only accepted answer is the non-negative root. That is:

\(\sqrt{9} = 3\), NOT +3 or -3;
\(\sqrt[4]{16} = 2\), NOT +2 or -2;

Notice that in contrast, the equation \(x^2 = 9\) has TWO solutions, +3 and -3. Because \(x^2 = 9\) means that \(x =-\sqrt{9}=-3\) or \(x=\sqrt{9}=3\).

No, the correct answer is still E.

From both statements we get the same: \(\frac{a + b}{\sqrt{ab}}=\frac{4b + b}{\sqrt{4b*b}}=\frac{5b}{\sqrt{4b^2}}=\frac{5b}{2|b|}=\) ?

If b is positive, then \(\frac{5b}{2|b|}=\frac{5b}{2b}=\frac{5}{2}\) (for example, plug b = 1)
If b is negatiive, then \(\frac{5b}{2|b|}=\frac{5b}{2(-b)}=-\frac{5}{2}\) (for example, plug b = -1)
If b is 0, then \(\frac{5b}{2|b|}=undefined\).

If sqrt cant give negative ans then why we are taking mod B. Its really confusing

Posted from my mobile device

Sqrt(4b^2) is 2b. Because in gmat sqrt cant be negative value

Posted from my mobile device

I tried explaining this above. |b| is an absolute value of b. An absolute value is always non-negative, so the square root does NOT produce negative result in this case too.