Is |x| + |x - 1| = 1? (1) x ≥ 0 (2) x ≤ 1

Can you please clarify as to how is \(x-x+1=1\) derived inB when it is provided that \(0\leq{x}\leq{1}\) that means X is +ve.


Thanks !!!

When \(x\) is in the range \(0\leq{x}\leq{1}\) then \(|x|=x\) and \(|x-1|=-(x-1)=-x+1\), thus \(|x|+|x-1|=1\) in this range becomes \(x-x+1=1\).

Hope it's clear.

I have got the answer as E. Can someone please clarify, what is wrong in my approach ?

Statement 1: X>=0

I assume X as 0
|0|+|0-1|= |0|+|1|= 1 ------------> Equation satisfied

I assume X as 3/4
|3/4|+|(3/4)-1|= |3/4|+|1/4|= 2 ----------> Equation unsatisfied

Statement 1 is not sufficient

Statement 2: X<=1

I assume X as 1
|1|+|1-1|= |1|+|0|= 1 --------------> Equation satisfied

I assume X as 3/4 again
|3/4|+|(3/4)-1|= |3/4|+|1/4|= 2 ----------> Equation unsatisfied

Statement 2 is not sufficient

Taking together both the equation, I assume X as 0, 3/4 and 1

When X=0, the answer is 1--------> Equation satisfied
When X=3/4, the answer is 2---------> Equation unsatisfied
When X=1, the answer is 1----------> Equation satisfied

Hence answer choice E is correct.

Clarifications needed, please.

l x l + lx-1l= 1 implies that sum of distance of x from 0 and from 1 should be 1. We can easily see that the distance between 0 and 1 is 1. So, x can lie on 0, on 1 or anywhere in between 0 and 1. It cannot go beyond 1 or before 0 because then the sum of the distance will become larger than 1.
Both statements together tell you that 0 <= x <= 1 and hence, the equation will hold for all such values of x.
Answer (C)

The correct answer is C, not E.

If x = 3/4, then Is |x| + |x-1| = |3/4| + |3/4 - 1| = 3/4 + 1/4 = 1.

Hope it helps.

My answer is C
its by plugging in method

1. From 1st equation , if x = 1 , then |x| + |x-1| = 1 , then its yes
if x = 5 , 9 # 1 , so No
so Equation 1 is No
2. From 2nd equation , of x = -1 , then its No
x = 1 its yes
so equation is No

3. using both , x = 0 then its Definite yes
So C..

Is my approach is Right

Using both, you get 0 <= x <= 1
x can lie anywhere from 0 to 1. Just trying the extreme values is not a good idea. You must try some values from the middle too i.e. x = 1/2, 1/4 etc.

Though establishing something by plugging in values is always tricky. You can try to negate a universal statement by looking for one value for which the statement doesn't hold but since you cannot try every value for which a statement must hold, its treacherous to depend on number plugging in this case. You should try to use logic.

Hi Karishma,

Please explain with an example how to find values for X when there is modulus in the equation.

For example |x-6| + |7-x| - | 4 - x| = |x + 2|

Thanks in Advance,
Rrsnathan.

The method stays the same though the thinking gets a little more complicated.

|x-6| + |7-x| - | 4 - x| = |x + 2|
|x-6| + |x - 7| = |x - 4| + |x + 2|

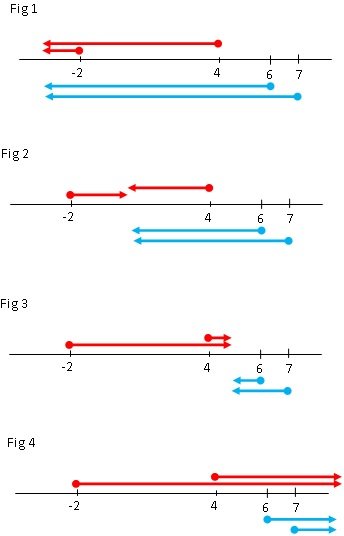
x is a point whose sum of distance from 6 and 7 is equal to sum of distance from 4 and -2.

Let's look at the various regions. We want the sum of lengths of red lines to be equal to sum of lengths of blue lines.

In Fig 1 (x lies to the left of -2), the sum of blue lines will always be greater than red lines since 6 and 7 are to the right so they will always need to cover extra distance.
In Fig 2 (x lies between -2 and 4), no matter where x is the sum of length of red lines is 6 units. Can the sum of blue lines be 6? Yes, from 7 to 4, one blue line covers 3 unit and from 6 to 4, the other blue line covers 2 units. Now both together need to cover 1 more unit so x will lie 0.5 unit to the left of 4 which gives the point 3.5.
In Fig 3 (x lies between 4 and 6 or between 6 and 7), the long red line will be at least 6 units and the maximum sum of blue lines can be 2 units + 3 units = 5 units. Hence sum of red lines will always be greater.
In Fig 4 (x lies to the right of 7), the red lines will always cover more distance than the blue lines since their points are to the left.

Hence, there is only one solution x = 3.5

Hi Bunuel,

Can you pls explain , for such kind of questions how do we decide what will be the check points ?

Like in your explanation, you said there are two check points 0 & 1 . Can you tell the approach behind this ?

Thanks in advance

Kunal

Check points or transition points are values of x for which expression in the modulus is 0. So, for |x| it's 0, for |x-1| it's 1, and for example for |2x-3| it's 3/2.

Theory on Abolute Values: math-absolute-value-modulus-86462.html
Absolute Value Tips: absolute-value-tips-and-hints-175002.html

DS Abolute Values Questions to practice: search.php?search_id=tag&tag_id=37
PS Abolute Values Questions to practice: search.php?search_id=tag&tag_id=58

Hope this helps.

Shouldn't this question be a DS question? This is the PS section.


You have two absolute values. The value inside the absolute values can be positive or negative. You can simplity to find all the values where |x| + |x-1| = 1. There are four possibilities. Positive-positive, positive-negative, negative-positive, and negative-negative.

x + x - 1 = 1. This will be 2x = 2 or x = 1.
x - 1 + 1 = 1. This will be x = 1.
-x + x - 1 = 1. This will be -1 = 1. This makes no sense, so you can ignore it.
-x - x + 1 = 1. This will be -2x = 0 or x = 0.

So according to the given formula, the only possible values of x that can result in 1 are when x = 1 or x = 0.

Statement 1: x >= 0. If x = 0 then true, but if x >1 then false. Insufficient.
Statement 2: x <= 1. If x = 1 then true, but if x<0 then false. Insufficient.
Statement 1 and 2: 0<=x<=1. Either way will make the statement true. Sufficient.

Bunuel. Hey abhimahna, could you please explain why we use this range \(0\leq{x}\leq{1}\) and not \(0\leq{x}<{1}\) in B (and \(x>1\) and not \(x\geq1\) in C)? If we look at this \(| x-1 |\), then won't that give us two ranges: x<1 & \(x\geq1\)?

From the GC Math book example, |x-1|=4, we get two ranges: 1) \((x-1)\geq0\) => \(x\geq1\) 2) (x-1)<0 => x<1.

Hey dabaobao ,

Yes, your point is completely valid.

But you need to understand that our question does contain other expressions other than |x-1|. Hence, we have to be very careful for such cases.

If you check the values, you will find that the expressions Bunuel has mentioned work fine.

Check out line method to solve such questions as explained by Karishma here.

I hope this helps.

|a-b| = the DISTANCE between a and b.

Thus:
|x| = |x-0| = the distance between x and 0.
|x-1| = the distance between x and 1.
|x| + |x-1| = the SUM of these two distances.

The distance between 0 and 1 is 1.

On the number line, if x is at or BETWEEN the two endpoints in blue, then the sum of the two distances will be EQUAL TO 1:
0 <--- |x| ---> x <---|x-1|---> 1
Here, |x| + |x-1| = the distance between 0 and 1 = 1.

By extension, if x is BEYOND either endpoint -- if x is to the left of 0 or to the right of 1 -- then the sum of the two distances will be GREATER THAN 1.

Thus, the answer to the question stem will be YES if 0≤x≤1.
Question stem, rephrased:
Is 0≤x≤1?

Clearly. each statement on its own is INSUFFICIENT.
When the statements are combined, we know that 0≤x≤1, so the answer to the rephrased question stem is YES.
SUFFICIENT.

Option (1) can be any number from 0 to infinity. hence, insufficient
Option (2) can mean any number from 1 to negative infinity. Hence, insufficient.

Taking them together, it means the number is between (and including ) 0 and 1. Take any value in between 0 and 1 (including both). 0.5, for example.

|x| = 0.5
|x-1| = |0.5-1| = |-0.5| = 0.5

Hence, |x| + |x-1| = 0.5 + 0.5 = 1

The same can be checked for other fractions between (and including) 0 and 1 as well and this equation will hold true.

Hence, option C

Remember the 12TEN mnemonic when answering DS questions!






Remember the mnemonic 12TEN for DS questions!

|x| + |x - 1| = 1 ?
|x + x - 1| = 1 ?
| 2x - 1| = 1?

A and B alone are not enough.
A and B combined = 0 <= x <= 1

If x = 0:
|2*0 - 1| = 1
If x = 1:
|2*1 - 1| = 1

However, nowhere in the question is it mentioned that x is an integer.
So if x = 0.75,

| 1.5 - 1 | equals 0.5

hence my answer will be E.